The Moon's 50-Day Cycle

By: James D. Dwyer
Date: August 3, 2013 +++
Copyright © 2016-2021 James D. Dwyer
Email: quest@creation-answers.com
Reference: www.design-of-time.com
You may freely copy, or distribute, this material
(Not to be sold)
_________________________________
Introduction
According to data published by the USNO, the average length of the synodic month is 29.530589 days, or also 29 days, 12 hours, 44 minutes, 3 seconds. The lunar period can thus be recognized to straddle a span of time that, on the average, terminates with the fractional part of a day.
The cited interface is especially puzzling because the length of the lunar month extends well beyond the boundary of a 24-hour day. This respective time cycle (of 29.530589 average days) then seems to reside outside any possible characteristic of purpose or design.
In a quest to understand a possible reason for the enigmatic period of the Moon, the current study looks at related information penned in ancient times by writers of the Bible. Some good news here is that a plausible day-count model of the Earth-Moon system is manifested from the content of various passages of Hebrew Scripture.
Subsequent pages and linked literature will then focus upon a model of the Moon that is outlined in biblical texts (and as is described in related literature).
Divisions within the lunar month
The Moon (as a shining object) mysteriously changes size and shape each night as it moves though cycles of waxing and waning. Of significance here is there are 4 distinct quarter phases of the Moon: 1.New phase; 2. First-quarter phase; 3. Full phase; and 4. Third-quarter phase.
The quarter phases are easy to recognize on the basis of observation. At the new phase the Moon is dark and appears to be completely invisible; at full phase, the Moon is fully-illuminated and is round-shaped; and at the first quarter and at the third quarter, the Moon is half illuminated and is distinctly divided into half-parts (half-light and half-dark, or the reverse).
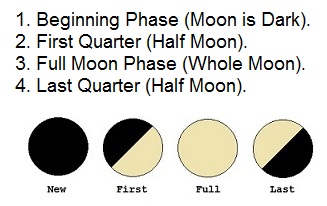
For the purposes of presenting a clear analysis, the cycle of the lunar quarter (which turns over every 7.38264725 days on the average) will hereafter be referred to as the cycle of the 'lunar week'.
As is further shown below, a perfect interface for the spin-orbits (of Earth and Moon) seems to be bound up in the traverse of time required by the lunar week.
A count of 50 days
Various passages of the Bible have detail of a scribe (or a count) of 7 weeks. Perhaps the best example of this time count can be recited from passages of Leviticus--where in Chapter 23 of the Hebrew version, a 7-weeks count is described to begin or to commence with 'mochorath h+shabbath' (which is presumed to mean the morrow of the Sabbath):
"Your scribe or number ('caphar') must extend from the morrow ('mochorath') to the Sabbath (h+shabbath) . . . " (Leviticus 23:15).
From this beginning or origin, a count that encompasses a time span equal to 7 whole weeks is shown: "7 Sabbaths shall be whole or entire". (Note here that the Hebrew Bible includes the word 'tamiym' to designate a week that is wholly or fully counted).
This passage of Scripture has further detail of the required presentation of a NEW ('chadesh') offering upon the next 'mochorath' (after 50 numbered days). The Bible text does thus describe a full or a complete count of 7 weeks followed by the celebration of a renewal day (with the presentation of an offering).
While Leviticus doesn't spell out that a 50-day scribe be predicated upon the phases of the Moon, writings produced by Flavius Josephus (a Jewish historian of the 1st century) are more specific in showing that Temple priests of that time were familiar with a Moon based count.
When describing a harvest calendar that was followed at the end of the Temple Era, Josephus made mention of a 50-day count traversing 7 lunar weeks--as follows:
" . . . when the Sun is in Aries . . . on the 16th day of the [lunar] month . . . they offer the first fruits of their barley . . . When a week of weeks has passed over after this sacrifice . . . on the 50th day, which is Pentecost . . . they bring to God [sacrifices] nor is there anyone of the [subsequent] festivals, but in it [= the 50th] they offer . . . " (Based upon Whiston's translation of 'Antiquities of the Jews', Book 3, Chapter 10, 5-7).
The Josephus record thus shows that the priests counted out a cycle of 7 weeks AFTER a barley offering was presented (on the 16th day of a specific lunar month). The cited 50-day count did therefore begin on a day that came after the full phase of the Moon.
One of the conclusions that can be arrived at from the detail provided by Josephus is that the end of the 50-day count would inherently have coincided with a quarter phase of the Moon. Furthermore, each of the intervening weeks of the 50-day count can be recognized to have passed in line with a lunar quarter. In essence, the priests can be recognized to have tracked a full cycle of 7 lunar weeks between the first fruits presentation and the feast of Pentecost.
So, the ancients appear to have understood the Bible's description of a 50-day count within the context of a count of 7 lunar weeks.
Many other passages of Hebrew literature have detail of a 50-day cycle. For more information, refer to the following online publication:
Annual count of time stations
As is further shown below, the above cited Levitical description of a renewal count (of 50 days) can ultimately be identified as one of the components of a day-count system that can effectively account for the two orbits (Sun and Moon).
The cited system that is effective for tracking the orbital returns surprisingly contains only one additional component. This component pertains to a month-like count that is likewise mentioned in a number of passages of Hebrew literature (including the Bible).
Of special significance here is an entire section of the book of Enoch (from chapter 71 to chapter 82) has a focus upon "the revolutions of the heavenly luminaries". (The cited portion of text that attempts to mathematically quantify the spin and orbital phenomenon is known as Enoch's astronomical book). Chapter 73, in particular, has detail of the simultaneous time track of a station of the Moon and also a station for the Sun:
[Chapter 71:] "The book of the revolutions of the luminaries of heaven, according to... their respective periods... and their respective months... according to every year of the world for ever... ." [Skipping to Chapter 73:] "... I beheld their stations... according to the fixed order of the months the Sun rises and sets... thirty days belonging to the Sun... [All the days belonging to each year can be correlated to a fixed number of days]... to the Sun and stars... thirty days belonging to them... The Moon brings on all the years exactly, that their stations may come neither too forwards nor too backwards a single day; but that the years may be changed with correct precision in [a fixed number of] days... The year then becomes truly complete according to the station of the Moon, and the station of the Sun... which rise and set in them for thirty days" (my paraphrase of the Laurence translation).
From the Enoch literature, it is apparent that the ancients did once time track a "station of the Sun"--probably in association with a cycle of 30 days. Portions of text from the astronomical book also make it clear that a "station to the Moon" was time tracked inside of the year cycle.
It here becomes most remarkable that "every year of the world forever" can effectively be determined by tracking nothing more than the two cited time stations (those described in Enoch's astronomical book)! Essentially, it is demonstrable that the rate of the solar year can effectively (perfectly!) be measured and metered out through the time track of a station (a day) of the Sun and a station (a day) of the Moon.
Through the continual reckoning of the two cited stations, the rate of the solar year can precisely (perfectly!) be correlated to a specific number of day units.
Hint: The rate of one day in each month-like cycle of 30 days is inherently equal to 12.17474 days per year (as an average rate). Also, the rate of one day in each cycle of 7 lunar weeks is inherently equal to 7.0676 days per year (on average). These two rates of days (as stations) are then equal to a composite rate 19.24232 days per year. Thus, as long as 19.24232 days or stations per year (on average) are reckoned apart from all other days then it becomes possible to determine the limits of each passing solar year in correspondence with a number count that is always equal to 346 of the other days. Note that the rate of the solar year of 365.24232 days minus the cited rate of stations (19.24232 days) is equal to 346 days.
It then seems clear that certain among the axioms and time formulas written down in Enoch's astronomical book are remarkably accurate. The solar circle (365.24219 days) inherently does contain a station or day of the Sun (one day in a 30-day cycle) and also a station or day of the Moon (one day in a cycle of 7 lunar weeks).
Thus, it seems significant to a study of Enoch's astronomical book that--as long as the cited stations of the Sun and Moon are routinely tracked apart from the other days--the length of each passing solar year is inherently metered into 346 equal divisions--on average. (Note that each of the cited 346 divisions inherently corresponds with the boundary of an exclusively counted day).
It is here very significant that the reckoning of 346 specific divisions (as exclusively counted days) results in a time span that is exactly equivalent to the length of the annual circle or year (in average time). Essentially, 346 days--when counted in association with 19.24232 renewal days per year--is equal to 365.24232 days. Thus, the annual result of routinely leaping the count of each station (or day) of the Sun and each station (or day) of the Moon is a time span that is exactly equal to the length of the annual circle or solar year (on the average). The average annual result of tracking 346 days in correspondence with stations of the Sun and Moon is perfect to within an annual difference of only 11 seconds! Remarkable is that (due to the slowing spin of the Earth) the annual result of tracking stations of the Sun and Moon can be recognized as fully or absolutely perfect relative to the rate of the solar year only several centuries before. For more specific information about accuracy inherent in tracking a station of the Sun and a station of the Moon, refer to the online publication:
An accounting of 346 annual stations (with the addition of stations of the Sun and Moon) is thus equivalent to an effective 'day-count' method for measuring and metering each tropical year.
The cited station of day of the Moon appears to have been accounted for at the frequency of every 7th lunar quarter (or every 7 lunar weeks). Of significance here is the required day or station of the Moon can perfectly keep pace with a running scribe of 50 days (but only as long as each station or day of the Sun is not included in the 50-day scribe). Thus, an accounting of 49 days (with the addition of stations of the Sun and Moon) can be be used as an effective 'day-count' method for ALSO measuring and metering the average passage of 7 lunar quarters. (More information about a scribe of 50 lunar days is shown below).
Divisions of the zodiac
Passages of text attributed to Enoch, and to other Jewish writers of the first century, reveal that the ancients would have been familiar with a method of charting 12 divisions of the zodiac.
Of significance here is that a simple math analysis proves that the ancients could have counted 173 world stations to perfectly measure and meter every half year cycle, and they could have counted 346 world stations to exactly determine the completion of every full year cycle.
Note here that days counted in association with the revolution of a station of the Sun (1 every 30 days) can be recognized to inherently occupy 3.33333 percent of time, and days counted in association with the revolution of a station of the Moon (1 day every 7 lunar quarters) can be recognized to occupy 1.93504 percent of time. So, if days corresponding to stations of the Sun and Moon are forever tracked then 5.26837 percent of time is inherently accounted for.
It then follows that if 'world stations' are assigned to represent a type, or class, of days (other than belonging within the domain of stations of the Sun and Moon) then this class of days can be recognized to inherently occupy 94.73163 percent of time [where this is the percent of time left over after 5.2684 percent of days are separated].
The genius behind separating a domain of days to occupy exactly 94.73163 percent of time can then be recognized from out of an accounting of 346 of those days.
The tropical year inherently occupies a time span equal to 365.24219 days. So, just how much of this time span is within the domain of 'world stations'? The answer, of course, is 94.73163 percent of the tropical year (which happens to be 345.99988 days).
The following diagram is consequently presented to more clearly illustrate that 4 seasonal divisions and 12 of the tropical zodiac can be cross-referenced to a count of 'world stations':
--------------------------------------------- A DAY-COUNT METHOD FOR TRACKING THE ZODIAC * --------------------------------------------- Season Quarter Zodiac Month Portal Number Day Month Days Day ------ ------- ------ -------- ------- 1 1 1 28 + 1 2 28 3 28 + 1 2 1 4 28 5 28 + 1 6 28 3 1 7 28 + 1 8 28 9 28 + 1 4 1 10 28 11 28 + 1 12 28 --------------------------------------------- 4 336 + 6 Year Total = 346 World Stations * -- This count equals 365.2423 days per year when paced by the addition of Sun and Moon stations.
It should be obvious from the rates expressed in the above diagram that an accounting of 346 'world stations' (with the addition of stations of the Sun and Moon) represents a very effective 'day-count' method for measuring and metering each tropical year.
As was previously noted, the modern count of 346 world stations comes to within only 11 seconds per year (on the average). What is remarkable about the accuracy inherent in tracking time stations is that, due to the slowing spin of the Earth, astronomers who were living in the relatively recent past would have been able to count out the year cycle to within the limits of perfect precision (at about 3 millennia ago). For more information about counting time stations, refer to the following online publication:
A Lunar Calendar
As was mentioned above, certain biblical passages show that period astronomers were familiar with a unique scribe of 50 days [= a scribe of 7 weeks plus 1 more day]. After 7 (full) weeks were accounted for, a renewal offering was then presented [= on the 'morrow of the 7th Sabbath'].
Of significance here is that a scribe of 50 days does inherently define a time span that is equal (on the average) with 7 lunar quarters, or 7 lunar weeks. However, this equivalency is only valid as long as the previously cited day or station of the Sun is not included in the days that are scribed.
The rate of 1 day in 30 days is inherently equal to 3.33333 percent of the time stream. This then means that a continual scribe of 50 days can closely pace the average return of a time cycle of 7 lunar quarters (of 51.678529 days). The 50-day scribe closely paces a cycle of 7 lunar weeks as long as 1 day in every 30 days is accounted for outside of, or in overplus to, a continual scribe of 50 days.
Please take note here that 7 lunar quarters (or 51.678529 days) minus 3.33333 percent of time is equal to 49.955911 days. However, a 50-day calendar can keep even more perfect pace with the revolution of 7 lunar quarters when the possibility of a 7-day festival is factored in. To be more specific about the scheduling of a festival, a fully accurate lunar calendar is possible by simply ignoring the addition of a 30th day at times when a festival is being celebrated. To better illustrate the possibility of achieving a more perfect lunar calendar, the following three possibilities for scheduling a festival are presented:
- Assuming a 7-day festival is held once each year then a surplus rate of 3.26945 percent of time is manifested from out of the overall strike rate of 30 days. This respective rate thus achieves 49.98893 average days versus a calendar count of 50 days.
- Assuming a 7 week festival is additionally held each 3rd year (as is further shown below) then a surplus rate of 3.24815 percent of time is manifested from out of the strike rate of 30 days. This respective rate thus achieves 49.99993 average days versus a calendar count of 50 days.
- Assuming one more day is additionally celebrated each 50th year (the jubilee year) then a surplus rate of 3.247971 percent of time is manifested from out of the strike rate of 30 days. This respective rate thus achieves 50.00002 average days versus a calendar count of 50 days.
Each passing lunar quarter can thus effectively (even perfectly) be measured and metered via a method of tracking a time station of the Sun (1 in a cycle of 30 days) and a time station of the Moon (1 day in a cycle of 7 lunar weeks).
---------------------------------------- A LUNAR-WEEKS CALENDAR * (50 days each pentecontad) ------ --------- -------------------- Lunar Number of Corresponding Weeks Week Days Pentecontad Days ------ --------- -------------------- 1 7 1 2 3 4 5 6 7 2 7 8 9 10 11 12 13 14 3 7 15 16 17 18 19 20 21 4 7 22 23 24 25 26 27 28 5 7 29 30 31 32 33 34 35 6 7 36 37 38 39 40 41 42 7 7 42 44 45 46 47 48 49 A 50th day is counted each 7th week ---------------------------------------- * - Requires the intercalation of a day of the Sun [= 1 in a 30 day cycle].
A weeks calendar (of 7 days each lunar quarter) can thus be kept into close pace with each average lunar quarter by the surplus accounting of a non-week day at each 30th day (of a 30-day cycle).
The surplus rate of 1 non-week day in 30 days is sufficient enough to closely keep a 50-day scribe aligned with each passing lunar quarter. However, a calendar that schedules a periodic festival would inherently be even more accurate. In fact, an absolutely perfect calendar is within the domain of leaping 30th days (as shown above).
A calendar of 50 years
The Scripture does furthermore have detail of not only a 50-day cycle but a 50-year cycle.
To be more specific, a requirement to release debtors each 50th year in a 50-year cycle is detailed in the 25th Chapter of the book of Leviticus--as follows:
"And the Lord said to Moses on Mount Sinai, Say to the children of Israel, When you come into the land which I will give you, let the land keep a Sabbath to the Lord. For six years put seed into your land, and for six years give care to your vines and get in the produce of them; But let the seventh year be a Sabbath of rest for the land, a Sabbath to the Lord; do not put seed into your land or have your vines cut. That which comes to growth of itself may not be cut, and the grapes of your uncared-for vines may not be taken off; let it be a year of rest for the land. And the Sabbath of the land will give food for you and your man-servant and your woman-servant and those working for payment, and for those of another country who are living among you; And for your cattle and the beasts on the land; all the natural increase of the land will be for food. And let seven Sabbaths of years be numbered to you, seven times seven years; even the days of seven Sabbaths of years, that is forty-nine years; Then let the loud horn be sounded far and wide at the tithe of the seventh [week] renewal; on the day of taking away sin let the horn be sounded through all your land. And let this fiftieth year be kept holy, and say publicly that everyone in the land is free from debt: it is the Jubilee, and every man may go back to his heritage and to his family. Let this fiftieth year be the Jubilee: no seed may be planted, and that which comes to growth of itself may not be cut, and the grapes may not be taken from the uncared-for vines. For it is the Jubilee, and it is holy to you; your food will be the natural increase of the field. In this year of Jubilee, let every man go back to his heritage." (my paraphrase of BBE text).
For additional information about the historic track of a cycle of 50 years, refer to the following online publication:
Of significance here is that Scroll 4QOtot shows the rotation of 24 courses of priests throughout a jubilee cycle (of 49 years).
Each course that is listed is by name the same as is listed in those Bible records that pertain to the First-Temple (under King Solomon). Josephus, who flourished late in the era of the Second-Temple, also mentioned that 24 courses of priests were rotated, and that each priestly course served the Temple for a term that lasted for only one week.
What is unique about the priests that are listed on Scroll 4QOtot is that they are shown to have been on duty at, or even prior, to the epoch of creation. The rotation of the Temple priests (24 courses) is thus shown to have been timeless (or endless) in that they are shown to have been on duty and serving in Heaven (well prior to the time Temple services were instituted by King Solomon).
Even more unique about the 'heavenly' priests that are listed on Scroll 4QOtot is that (throughout the rotation of their 24 courses) a lunar-cycle 'sign' appears to have been reckoned (at a continuous frequency of each 3 years). Also unique is that each cycle of 7 years, and each jubilee cycle of 49 years, appears to have been endlessly accounted for.
This mystic depiction of 24 courses of priests performing unending services in pace with a 7-day cycle, a 3-year cycle, a 7-year cycle, and a 49-year cycle is puzzling in that 4 diverse time units are referenced.
Remarkable here is that the various cycles that are listed (on Scroll 4Qotot) can all be recognized to be elements of an effective time-tracking system (when all are brought under the lens of astronomy).
As is shown in the subsequent diagram, a jubilee calendar becomes the inherent, or the automatic, result of simply skipping the count of a lunar week each and every 3rd year:
---------------------------------------- A JUBILEE CALENDAR OF LUNAR WEEKS ----------------------- -------------- Year 1: 49 weeks Year 8: 49 weeks Year 2: 49 weeks Year 9: 49 weeks Year 3: 49 weeks Year 10: 49 weeks Year 4: 49 weeks Year 11: 49 weeks Year 5: 49 weeks Year 12: 49 weeks Year 6: 49 weeks Year 13: 49 weeks Year 7: 49 weeks Year 14: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 15: 49 weeks Year 22: 49 weeks Year 16: 49 weeks Year 23: 49 weeks Year 17: 49 weeks Year 24: 49 weeks Year 18: 49 weeks Year 25: 49 weeks Year 19: 49 weeks Year 26: 49 weeks Year 20: 49 weeks Year 27: 49 weeks Year 21: 49 weeks Year 28: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 29: 49 weeks Year 36: 49 weeks Year 30: 49 weeks Year 37: 49 weeks Year 31: 49 weeks Year 38: 49 weeks Year 32: 49 weeks Year 39: 49 weeks Year 33: 49 weeks Year 40: 49 weeks Year 34: 49 weeks Year 41: 49 weeks Year 35: 49 weeks Year 42: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 43: 49 weeks Year 44: 49 weeks Year 45: 49 weeks Year 46: 49 weeks Year 47: 49 weeks Year 48: 49 weeks Year 49: 49 weeks At 7th Year: 1 week ------------------- Year 50: 49 weeks
Take note that in order to keep pace with the turn of each tropical year, the diagrammed calendar requires the addition of a lunar week each 3rd year (a perpetual rate).
Of significance about the shown jubilee calendar is that with the stated rate of required intercalation applied, each calendar year--on the average--becomes equal to 365.2442 days. Each year of the cited jubilee calendar then compares very closely with the revolution of the tropical year--which rolls over in 365.2422 days. The jubilee calendar (as diagrammed) thus depicts a time cycle (in years) that can effectively be measured and metered out in association with a number of lunar weeks (or lunar quarters).
It should be clear from the week counts shown in the diagram that--when the rate of one lunar week every 3rd year is counted apart (or leaped) from out of the time stream--a grid of lunar weeks (2457 quarters) can be counted (repeated) in correspondence with a cycle of 50 years. Essentially, an effective calendar of lunar weeks is the inherent or automatic result of leaping one week each 3rd year from out of the time stream. (This respective rate of calendar intercalation is equivalent to 0.33333 weeks per solar year on the average).
Thus, it becomes of considerable significance to a study of interrelated time design that an effective annual calendar is the inherent result of counting lunar weeks.
The above shown calendar of lunar weeks would inherently remain accurate relative to the pace of the tropical year over many centuries of time. The time difference between the respective 49-week calendar and the length of the solar year (which turns every 365.2422 days) would eventually become a factor if enough time were to pass by. To be specific, assume that a new phase of the Moon was observed (as the first day of the calendar) at say 7 days prior to the day of the vernal equinox. From this origin and alignment, the first day of the calendar would inherently shift (on average) from year to year so that after 3600 years the first calendar day would arrive in alignment with the equinox, and after 7200 years the first calendar day would come 7 days after the equinox. Somewhat remarkable here is that the Bible (and associated records) DO point to a literal epoch day for the creation [= right at 7 days prior to the day of the vernal equinox]. For more information about Creation's epoch day, refer to:
While Scroll 4QOtot doesn't explicitly show that a lunar week was specially accounted for at the distance of each 3rd year, it seems very clear that the heavenly priests were believed to have perpetually reckoned a lunar-cycle 'sign' at this respective distance (each and every 3 years). This leaves some latitude in interpreting how the lunar cycle was once reckoned. For example, in reckoning the 'sign', the priests may have reckoned the lunar cycle at the resolution of the half or the whole of the lunar cycle.
The main reason for believing that the lunar cycle was once reckoned at the resolution of the quarter phase is that ancient literature is explicit in describing the priestly courses as being rotated once each week. The routinely appearing 'sign' was then accounted for right when one priestly course ended (refer to Scroll 4QOtot). The combination of this rotating schedule and the time when the 'sign' was routinely observed does not seem to allow for an alternate interpretation. Essentially, if the 'sign' was observed at the end of a 'week' cycle then it is obvious that the priests were reckoning lunar weeks.
The indicated track of a lunar 'sign' points to the possibility that the priests recognized certain among the lunar weeks to be very special. The respective week which corresponded to the lunar 'sign' was apparently not counted the same as were other calendar weeks.
Somewhat puzzling about the jubilee cycle shown on Scroll 4QOtot is that a jubilee cycle of 49 years is listed while Leviticus (Chapter 25) shows the addition of a 50th year (throughout which the jubilee year was celebrated).
In terms of astronomy and of accuracy, a calendar of lunar weeks (a 50-year calendar) is automatic or inherent when a lunar week is leaped each 3rd year as a perpetual rate. (The cited grid of lunar weeks very, very closely paces the rate of the solar year through the intercalation of 0.33333 weeks per solar year--as an average rate).
Thus, a given conclusion from the 'lunar sign' is that a jubilee cycle (of 50 full years) can be cross-referenced to a calendar of lunar weeks. This remarkable lunisolar cross-reference is easy to recognize when a lunar week is perpetually intercalated each 3rd year.
It is possible that the indicated 'sign' does in someway relate to an early used tithing cycle. However, a more easy to recognize reason is that the 'sign' was tracked across 3 years in tandem with the renewal of 30 days. For information about a biblically described tithe in the 3rd year, refer to the following publication:
For more information about early priests and ancient astronomy, refer to the following online publications:
_________________________________
<End Of Document>