The Moon as a Time Meter

________________________________
By: A-Quest-for-Creation-Answers
Revision/Update: October 20, 2012 +++
Copyright © 2007-2021 James D. Dwyer
Email: quest@creation-answers.com
Reference: www.design-of-time.com
You may freely copy, or distribute, this material
(Not to be sold)
________________________________
Introduction
Passages written within the Bible inform us that the Earth, Moon and Sun are the result of a special creation. To be more specific, Bible texts relate that a primary function of the Sun and Moon is to provide luminance. Day (divided from Night) is shown in a time sequence ruled by the Sun, while Night is depicted in a time sequence governed by the Moon.
According to the author (or authors) of the book of Genesis, the movement of the Sun and Moon generates not only 'signs', but also 'fixed times', and cycles of days and years [= Hebrew: owth, mow`ed, yowm, and shaneh].
Subsequently presented paragraphs and linked literature will attempt to show that the writers of the Bible cannot be completely ignored. In fact, the orbital rates of the Earth and Moon can be recognized to ALL clock together so as to divide the time stream into a functional arrangement.
A lunar 'sign'
In early Israel, a span of 7 years was used to compute various long time cycles. One of these long time cycles was a span of 7 sets of 7 years (or 49 years). After 49 years were counted-out, a special jubilee celebration was held to announce the commencement of the jubilee year (or the 50th year).
The content of certain early-written manuscripts reveals that the jubilee year may have been celebrated in association with a system of lunar reckoning. As an example, Scroll 4QOtot is explicit in showing the routine occurrence of a lunar-cycle 'sign' in association with a count of the jubilee cycle. (The priests when revolving their courses throughout the jubilee time cycle appear to have reckoned a lunar-cycle 'sign' at a continuous frequency of each 3 years).
As is shown below, astronomy makes it more certain that if the priests had observed a lunar-cycle 'sign' (at the frequency or rate of each and every 3rd year) then the priests might have been observing one of the quarter phases of the Moon. It seems that the boundary of a quarter phase of the Moon does literally revolve into almost perfect alignment with the boundary of each 3rd year.
Note: There are 4 distinct quarter phases of the Moon: 1.New phase; 2. First-quarter phase; 3. Full phase; and 4. Third-quarter phase. The quarter phases are easy to recognize on the basis of observation. At the new phase the Moon is dark and appears to be completely invisible; at full phase, the Moon is fully-illuminated and is round-shaped; and at the first quarter and at the third quarter, the Moon is half illuminated and is distinctly divided into half-parts (half-light and half-dark, or the reverse).
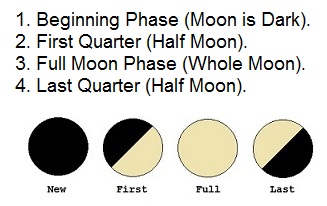
For the purposes of presenting a clear analysis, the lunar quarter (which completes in 7.38 days) will hereafter be referred to as a 'lunar week'.
Of interest about the content of Scroll 4QOtot is that 24 courses of Temple priests are shown to have revolved or rotated their respective courses throughout a jubilee cycle (of 49 years).
Each course that is listed is by name the same as is listed in those Bible records that pertain to the First-Temple (under King Solomon). Josephus, who flourished late in the era of the Second-Temple, also mentioned that 24 courses of priests were rotated, and that each priestly course served the Temple for a term that lasted for only one week.
What is unique about the priests that are listed on Scroll 4QOtot is that they are shown to have been on duty at, or even prior, to the epoch of creation. The rotation of the Temple priests (24 courses) is thus shown to have been timeless (or endless) in that they are shown to have been on duty and serving in Heaven (well prior to the time Temple services were instituted by King Solomon). A number of 24 officers (or elders) are likewise shown sitting on heavenly thrones in diverse passages of the book of Revelation.
Even more unique about the 'Heavenly Priests' that are listed on Scroll 4QOtot is that (throughout the rotation of their 24 courses) a lunar-cycle 'sign' appears to have been reckoned (at a continuous frequency of each 3 years). Also unique is that each cycle of 7 years, and each jubilee cycle of 49 years, appears to have been endlessly accounted for.
This mystic depiction of 24 courses of priests performing unending services in pace with a 7-day cycle, a 3-year cycle, a 7-year cycle, and a 49-year cycle is puzzling in that 4 diverse time units are referenced.
Remarkable here is that the various cycles that are listed (on Scroll 4Qotot) can all be recognized to be elements of an effective time-tracking system (when all are brought under the lens of astronomy).
As is shown in the subsequent diagram, a jubilee calendar becomes the inherent, or the automatic, result of simply skipping the count of a lunar week each and every 3rd year:
---------------------------------------- A JUBILEE CALENDAR OF LUNAR WEEKS ----------------------- -------------- Year 1: 49 weeks Year 8: 49 weeks Year 2: 49 weeks Year 9: 49 weeks Year 3: 49 weeks Year 10: 49 weeks Year 4: 49 weeks Year 11: 49 weeks Year 5: 49 weeks Year 12: 49 weeks Year 6: 49 weeks Year 13: 49 weeks Year 7: 49 weeks Year 14: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 15: 49 weeks Year 22: 49 weeks Year 16: 49 weeks Year 23: 49 weeks Year 17: 49 weeks Year 24: 49 weeks Year 18: 49 weeks Year 25: 49 weeks Year 19: 49 weeks Year 26: 49 weeks Year 20: 49 weeks Year 27: 49 weeks Year 21: 49 weeks Year 28: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 29: 49 weeks Year 36: 49 weeks Year 30: 49 weeks Year 37: 49 weeks Year 31: 49 weeks Year 38: 49 weeks Year 32: 49 weeks Year 39: 49 weeks Year 33: 49 weeks Year 40: 49 weeks Year 34: 49 weeks Year 41: 49 weeks Year 35: 49 weeks Year 42: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 43: 49 weeks Year 44: 49 weeks Year 45: 49 weeks Year 46: 49 weeks Year 47: 49 weeks Year 48: 49 weeks Year 49: 49 weeks At 7th Year: 1 week ------------------- Year 50: 49 weeks
Take note that in order to keep pace with the turn of each tropical year, the diagrammed calendar requires the addition of a lunar week each 3rd year (a perpetual rate).
Of significance about the shown jubilee calendar is that with the stated rate of required intercalation applied, each calendar year--on the average--becomes equal to 365.2442 days. Each year of the cited jubilee calendar then compares very closely with the revolution of the tropical year--which rolls over in 365.2422 days. The jubilee calendar (as diagrammed) thus depicts a time cycle (in years) that can effectively be measured and metered out in association with a number of lunar weeks (or lunar quarters).
It should be clear from the week counts shown in the diagram that--when the rate of one lunar week every 3rd year is counted apart (or leaped) from out of the time stream--a grid of lunar weeks (2457 weeks) can be counted (repeated) in correspondence with a cycle of 50 years. Essentially, an effective calendar of lunar weeks is the inherent or automatic result of leaping one week each 3rd year from out of the time stream. (This respective rate of calendar intercalation is equivalent to 0.33333 weeks per solar year on the average).
Thus, it becomes of considerable significance to a study of interrelated time design that an effective annual calendar is the inherent result of counting lunar weeks.
The above shown calendar of lunar weeks would inherently remain accurate relative to the pace of the tropical year over many centuries of time. The time difference between the respective 49-week calendar and the length of the solar year (which turns every 365.2422 days) would eventually become a factor if enough time were to pass by. To be specific, assume that a new phase of the Moon was observed (as the first day of the calendar) at say 7 days prior to the day of the vernal equinox. From this origin and alignment, the first day of the calendar would inherently shift (on average) from year to year so that after 3600 years the first calendar day would arrive in alignment with the equinox, and after 7200 years the first calendar day would come 7 days after the equinox. Somewhat remarkable here is that the Bible (and associated records) DO point to a literal epoch day for the creation [= right at 7 days prior to the day of the vernal equinox]. For more information about Creation's epoch day, refer to:
While Scroll 4QOtot doesn't explicitly show that a lunar week was specially accounted for at the distance of each 3rd year, it seems very clear that the heavenly priests were believed to have perpetually reckoned a lunar-cycle 'sign' at this respective distance (each and every 3 years). This leaves some latitude in interpreting how the lunar cycle was once reckoned. For example, in reckoning the 'sign', the priests may have reckoned the lunar cycle at the resolution of the half or the whole of the lunar cycle.
The main reason for believing that the lunar cycle was once reckoned at the resolution of the quarter phase is that ancient literature is explicit in describing the priestly courses as being rotated once each week. The routinely appearing 'sign' was then accounted for right when one priestly course ended (refer to Scroll 4QOtot). The combination of this rotating schedule and the time when the 'sign' was routinely observed does not seem to allow for an alternate interpretation. Essentially, if the 'sign' was observed at the end of a 'week' cycle then it is obvious that the priests were reckoning lunar weeks.
For pertinent information confirming that Temple priests did once track lunar-quarters or lunar weeks, refer to the following online publication:
The indicated track of a lunar 'sign' points to the possibility that the priests recognized certain among the lunar weeks to be very special. The respective week which corresponded to the lunar 'sign' was apparently not counted the same as were other calendar weeks.
Note that a leap week occurring each 3rd year is not shown in the following calendar chart.
______________________________________ 7 sets of 7 years can be defined by counting 7 sets of 7 lunar weeks ______________________________________ 7-Yr No. Number of At Each Seg Yrs Lunar Weeks 7th Year ---- --- ----------------- -------- 1. 7 7 times 7 times 7 + 1 week 2. 7 7 times 7 times 7 + 1 week 3. 7 7 times 7 times 7 + 1 week 4. 7 7 times 7 times 7 + 1 week 5. 7 7 times 7 times 7 + 1 week 6. 7 7 times 7 times 7 + 1 week 7. 7 7 times 7 times 7 + 1 week ---- --- ----------------- -------- 50th = 1 yr 7 times 7 ______________________________________ Note that a leap week (a 3-year rate) is required to keep the depicted 7 sets into alighment with 7 sets of years.
The diagram shown above is synonymous to the previous diagram in showing that primal priests may have tracked lunar phases to effectively track the limits of a 50-year cycle.
Somewhat puzzling about the jubilee cycle shown on Scroll 4QOtot is that a jubilee cycle of 49 years is listed while Leviticus (Chapter 25) shows the addition of a 50th year (throughout which the jubilee year was celebrated).
In terms of astronomy and of accuracy, a calendar of lunar weeks (a 50-year calendar) is automatic or inherent when a lunar week is leaped each 3rd year as a perpetual rate. (The cited grid of lunar weeks very, very closely paces the rate of the solar year through the intercalation of 0.33333 weeks per solar year--as an average rate).
Thus, a given conclusion from the 'lunar sign' is that the biblical jubilee cycle (of 50 years) can be cross-referenced to a calendar of lunar weeks. This remarkable lunisolar cross-reference is easy to recognize when a lunar week is perpetually intercalated each 3rd year.
It is possible that the indicated 'sign' does in someway relate to an early used tithing cycle. However, a more easy to recognize reason is that the 'sign' was tracked across 3 years in tandem with the renewal of 30 days.
For pertinent information about the interpretation of a tithe in the 3rd year, refer to the following publication:
For more comprehensive information concerning the once observed jubilee cycle, refer to the following online publications:
A cycle of 49 lunar months
What is remarkable about tracking the jubilee cycle is an inherent correspondence with sets of lunar weeks that line up (or interface) with multiples of sevens. (For more information about counting lunar weeks in '7 sets', refer to the previously presented section).
A very obvious instance of the Moon's conformance with '7-set design' is that the orbital return--when clocked in terms of Earth's rotation--does precisely define a long cycle of 49 months. Of significance about the turn over of 49 months is that the Earth and the Moon do both renew together right at the same hour and minute (on average). This alignment of the solar day with the lunar month is just about perfect.
Thus, a given conclusion from this rotational alignment of the Earth with the Moon is that a span of time equal to 49 moons can exactly be divided into solar-day units (where each solar day is equal to 24 hours, or also 86400 seconds).
Take note here that the lunar month (of 29 days 12 hours, and 44 minutes on the average) if repeated for 49 times does inherently traverse a time span that is almost perfectly equal to 1447 rotations of the Earth (when the rotational rate is measured in terms of solar days).
____________________________________ THE INTERFACE OF 49 SYNODIC MONTHS ____________________________________ Number of Number of Months * Earth's Rotations --------------------------- ------- 1 2 3 4 5 6 7 206.71 8 9 10 11 12 13 14 413.43 15 16 17 18 19 20 21 620.14 22 23 24 25 26 27 28 826.86 29 30 31 32 33 34 35 1033.57 36 37 38 39 40 41 42 1240.28 43 44 45 46 47 48 49 1447.00 --------------------------- ------- * - A span of 49 lunar months is equal to 1447 days.
Thus, it would be a true axiom to state that 49 lunar months (on the average) are equal to a time span that can be divided into an even number of 24-hour days.
It would likewise be a true axiom to state that 1447 days is equal to a time span that can be divided into an even number of lunar months.
The definition of a conjunction cycle between the Earth and the Moon every 49 months is easy for almost anyone to prove. This relationship can be demonstrated by simply dividing 1447 (days) by 49. The result of the division can then be compared with the span of time occupied by the average lunar month--which is 29.53059 days. Note that a correct answer from performing this math will not differ from 29.53059 by more than 2 seconds!!!
The cited synchronization of Earth's spin with 49 lunar months is very close (almost exact). Of significance here is that the stated interface can be recognized as fully perfect if only the lunar period elapsed in 29.53061 days (which is 2 seconds different from the modern rate of 29.53059 days). The possibility then is that--due to tiny variations in the rate of Earth's spin--the conjunction of Earth's spin with the lunar period may have once been fully perfect. For more information, refer to:
A cycle of 7 lunar weeks
From the perspective of a resident living on the Earth, a calendar comprised of lunar quarters would not be as convenient to use as a more traditional calendar that accounts for time by months and days. As is further shown below, the tropical year CAN quite perfectly be correlated to a fixed number of days. Of special interest about 'day counting' the year cycle is that a collection of Hebrew axioms and formulas for resolving the courses of the Earth and Moon are available for modern analysis. This ancient collection is represented in passages of a rediscovered manuscript attributed to Enoch (one of the Bible patriarchs). In fact, an entire section of the Enoch literature (from chapter 71 to chapter 82) has a focus upon "the revolutions of the heavenly luminaries". (The cited portion of text that attempts to mathematically quantify the spin and orbital phenomenon is known as Enoch's astronomical book).
The content of the collection attributed to Enoch is unique in that a rather comprehensive description of tracking 'time stations' is embedded in the astronomical section.
Early-held knowledge of the location of time stations for both the Sun and the Moon seems very apparent from the following selected portions of 'The Ethiopian Enoch', by Laurence:
[Chapter 71:] "The book of the revolutions of the luminaries of heaven, according to . . . their respective periods . . . and their respective months . . . [Skipping to Chapter 73:] . . . I beheld their stations . . . according to the fixed order of the months the Sun rises and sets . . . thirty days belonging to the Sun . . . The Moon brings on all the years exactly, that their stations may come neither too forwards nor too backwards a single day; but that the years may be changed with correct precision . . . The year then becomes truly complete according to the station of the Moon . . . ".
From the Enoch literature, it is apparent that the ancients did once time track a "station" of the Sun--probably in association with a cycle of 30 days. Portions of text from the astronomical book also make it clear that a "station to the Moon" was time tracked inside of the year cycle. In essence, in addition to a station of the Sun, Enoch's astronomical book also describes an associated station of the Moon.
"The year then becomes truly complete according to the station of the Moon, and the station of the Sun" (ibid.).
According to the astronomical book, in addition to a station of the Sun, a station of the Moon also belongs among (pertains to) the revolutions of the heavenly luminaries.
Thus, the detail given for time stations indicates that some among the ancients held knowledge of an effective method for tracking each annual return (the year cycle). Of significance here is that Enoch's axiom for metering the year cycle was stated only in terms of the revolution of two time stations:
- A day or station defined by the Moon.
- A day or station defined by the Sun.
Of additional significance is that other portions of the Enoch literature indicate the cited station or day of the Moon might have been tracked in place, or in position, with a sequence of the lunar quarters. This positioning of a station or day of the Moon in correspondence with a cycle of the lunar-quarter phases is easy to interpret from the following portions of the cited astronomical book:
"(Chapter 72: verse 3) . . . [the Moon's] light is a seventh portion from the light of the Sun . . . . (verse 6) Half of it is in extent seven portions . . . its light is by sevens . . . (verse 8-10) On that night, when it commences its period . . . it is dark in its fourteen portions . . . During the remainder of its period its light increases to fourteen portions [or the Moon's light increases to fourteen portions] . . . (Chapter 73: verse 4) In each of its two seven portions it completes all its light [or the Moon reaches the phase of full illumination in two seven portions] ." (ibid.).
A more in depth research of Enoch's astronomical book leads to the ultimate conclusion that the cited station or day of the Moon was probably tracked in association with a cycle of 7 lunar quarters or 7 lunar weeks. The clue to coming up with a more explicit definition of the station of the Moon from the astronomical book can seemingly be found in Chapter 73 in the portion of text that provides detail of the Moon and its lag of 50 days. ("To the Moon alone . . . it has fifty days . . . ").
It can thus ultimately be interpreted that primal priest-astronomers did once reckon lunar weeks and were knowledgeable of a station or day of the Moon (in addition to the cited station of the Sun). The station of the Moon appears to have been tracked in correspondence with a time-span of 7 lunar quarters or 7 lunar weeks.
The description of a station or a day of the Moon from the Enoch texts is then significant and tends to indicate the early use of the following axiom or time formula:
The revolutions of the heavenly luminaries define a station or day that pertains to the Moon. This station or day reoccurs in a cycle of 7 lunar weeks (an endless rate).
Of significance here is that each year cycle (year . . . after year . . . after year . . . ) can be correlated to a day count that does never vary as long as those days that reoccur in the position of each 7th lunar week are leaped over (or are not counted).
Note that if the count of one day in each cycle of 7 lunar weeks is eternally accounted for (as separate from the other days) then this respective count is inherently equal to 7.0676 days per year. In addition, if the count of one day in each month of 30 days is forever accounted for (as separate from other days) then this respective count is inherently equal to 12.17474 days per year (as an average rate). These two rates of set-apart days (or time stations) are then equal to an average rate of 19.24232 days per year. Thus, if 19.24232 days per year (on the average) are tracked apart from all other days that comprise the time stream then the length of each passing solar year can effectively be measured and metered out in correspondence with a number count that is always equal to 346.000 of the other days.
It is then clear that the turn of each tropical year can exactly be defined (as an average definition) in the context of nothing more than forever tracking a station of the Sun (each 30th day) and also eternally tracking a station of the Moon (at every 7th lunar week). In essence, within the context of both monthly and weekly renewals, each passing tropical year (which is 365.24 days in length) can be understood to revolve in perfect pace with an identical count of day units (346 days). To be completely specific, an accounting of 346 days with the addition of renewal days (19.24 days) is inherently equal to the length of the annual circle or year.
Thus, certain among the axioms and time formulas written down in Enoch's astronomical book are proven as remarkably accurate. The solar circle (365.24219 days) inherently does contain a station or day of the Sun (a perpetual rate of one in a 30-day cycle) and also a station or day of the Moon (a perpetual rate of one in a cycle of 7 lunar weeks).
_________________________________ ENDLESS CYCLE OF 7 LUNAR WEEKS _________________________________ Lunar quarter 1 (lunar week 1) Lunar quarter 2 (lunar week 2) Lunar quarter 3 (lunar week 3) Lunar quarter 4 (lunar week 4) Lunar quarter 5 (lunar week 5) Lunar quarter 6 (lunar week 6) Lunar quarter 7 (lunar week 7) _________________________________
Especially significant about the astronomy of Enoch is the revelation of a day-count model that can exactly account for each passing tropical year. (This accounting of the year cycle only requires a separated time track of Sun and Moon stations). For more information about time stations in history, refer to the following online publications:
'30 days' as a calendar count
As was outlined within the previous section, a continuous (unbroken) count of 30 days is huge to a study of interrelated time design. In fact, each passing tropical year (of 365.24219 days) can precisely be measured to within the limits of only 11 seconds (by keeping track of each 30th day).
What is even more remarkable is that it is possible to even more precisely define each tropical year by using only a cycle of 30 days as a clock (or a meter).
Of significance here is that an incredibly precise solar calendar can be derived from out of the rate of a primary time cycle that spans 360 days.
The following diagram shows that the time traverse of each tropical year can effectively be measured and metered out by tracking primary and secondary cycles of 360 days -- where the secondary cycle represents a calendar count that is preempted by the inclusion of additional days:
_____________________________________ A PERFECT 9-YEAR CALENDAR * _____________________________________ Renewal = 1 day Year 1 = 360 days Year 2 = 360 days Year 3 = 360 days Year 4 = 360 days Year 5 = 360 days Year 6 = 360 days Year 7 = 360 days Year 8 = 360 days Year 9 = 360 days * - 5 days are added every 360 days and 1 more day is added to years containing a 5th quarter node. ____________________________________ Average calendar rate = 365.24217 days Solar-year rate = 365.24219 days Average difference = 2 seconds (!)
For specific technical information about a calendar count of 360 days, refer to the following online publication:
Remarkable about a time wheel of 360 days is that an accounting of only two short cycles can achieve what is probably the very most accurate calendar that can be derived from out of the spin and orbital phenomenon.
A time cycle of 360 days can also be recognized from various passages of the Bible. This time unit is implicit from the Genesis record of the flood, and a cycle of 360 days is also shown in passages of Revelation.
For information of 360 days in the Bible record, refer to the following online publications:
Of significance here is that several of the old-world calendars appear to have been predicated upon the revolution of 360 days.
A cycle of 16 years
Some among the ancient writers left record of various long cycles (of years). Certain of these year counts can ultimately be recognized to represent a twofold track of the Moon and Sun.
Of significance here is that several among the Jewish and Christian astronomers--those who flourished within the first 4 centuries (CE)--can be recited to have been familiar with more than a single lunisolar system.
One lunisolar cycle that early astronomers would have been familiar with was the Metonic Cycle (named for an Athens astronomer). This cycle is 19 years in length, and it is defined by a somewhat close conjunction of 235 lunar periods with 19 tropical years. (Note here that 235 synodic months are equal to 6939.69 days and 19 solar years are equal to 6939.60 days). The time span occupied by 235 lunar months is--however--longer than 19 years by an amount of over 2 hours.
The early use of the Metonic Cycle by a Christian astronomer can be recited from the writings of an early writer named Anatolius. In fact, this respective author wrote out a comprehensive set of instruction by which the Moon can be charted across a time span of 19 years.
In his Pascal Canon, Anatolius additionally noted that certain contemporary Christians were then familiar with different (perhaps even more accurate) lunisolar systems. He described the following use of 4 other methods by which lunar and solar time cycles were currently being tracked--as follows:
"... on the subject of the order of the times... no mode of computation is to be approved, in which... [the courses of the Sun and the Moon] are not found together... in the books of the Hebrews and Greeks, we find not only the course of the Moon, but also that of the Sun, and, indeed, not simply its course in the general, but even the separate and minutest moments of its hours all calculated... Of these Hippolytus made up a period of 16 years with certain unknown courses of the Moon. Others have reckoned by a period of 25 years, others by 30, and some by 84 years... "(refer to 'The Paschal Canon of Anatolius', translated by Salmond).
Thus, in addition to providing detail of a cycle of 19 years, Anatolius also cited that contemporary astronomers held knowledge of other lunisolar systems (of 16 years, of 25 years, of 30 years, and of 84 years).
This mention of a period of 25 years can possibly be identified from amid the writings of Saint Jerome. For more information about a time track of 25 years, click on the following link:
As far as a time count of 84 years, this cycle may have been predicated upon a time count of 40 days. (Note here that 84 years contains 30680 days or also 767 cycles of 40 days). For more information about a calendar counnt of 40 days:
The above citation of a time period that lasted for 30 years was probably made in reference to an early lunar calendar that charted 371 months across 30 years. A calendar of this type was predicated upon half-month cycles, and appears to have been popular among the Gauls. For more information, try researching the Coligny Calendar from numerous sources that are available on the Internet.
Last, but not least, if the above stated period of 16 years is brought under the lens of modern astronomy--and along side of a law attributed to Enoch--then a number of details surrounding of an incredibly accurate lunisolar system begin to become recognizable.
In assembling the historic data all together, one piece of possibly pertinent text can be extracted from out of the Enoch literature. This particular portion of text almost strangely compares the Moon to, or within, the limits of 8 years--as follows:
"And I saw another [time] course, a law for her [= the Moon], how . . in 8 years there are . . . days. For the moon alone the days . . . in 8 years amount . . . all the days . . . in 8 years . . . ".
The stated course of the Moon is significant in regard that if the synodic return of the Moon is routinely accounted for in half-day units then an effective (perfect!) time track of the limits of 8 tropical years can ultimately be achieved.
In connecting one piece of information with the next, the cited time course of 8 years would probably have been understood among the ancients as also being synonymous with a fuller or longer count of 16 years. Note here the 8-year law for the Moon--when stated in terms of whole days--inherently becomes equal to a double cycle of 8 tropical years, or is equal to a span of 16 tropical years.
Then, to more clearly illustrate how primitive astronomers might have computed the return of the Moon, passages of early-written text pertaining to Hippolytus become the more pertinent:
". . . Hippolytus made up a period of 16 years with certain unknown courses of the Moon" ('The Paschal Canon').
From other passages of early-written literature, it can be recognized from the cited reference to Hippolytus that this early astronomer was both a learned scholar and a prolific writer. As a Christian theologian, he became rather influential at Rome.
Unfortunately, for reasons that are not completely clear, Hippolytus was either murdered, or he suffered martyrdom. (His death occurred in or about the year 238 CE).
Based upon lunisolar accounting attributed to Hippolytus, it is clear that this ancient astronomer was also familiar with a long cycle of 112 years (refer to Easter Controversy at the New Advent web site on the Internet).
Hippolytus is thus shown to have possessed the means of predicting the spin and orbital phenomenon in association with a long cycle comprised of both 16 years and 112 years. (Note that the stated great cycle of 112 years was almost surely time tracked in 7 segments of 16 years each).
What is significant concerning the stated great cycle of 112 years (and similar information contained in ancient "books of the Hebrews and Greeks") is that the spin and orbital rates (Earth and Moon) can be demonstrated to exactly keep pace with a time grid comprised of 16-year segments.
To be more specific, it would be a true axiom to state that if the rate of the synodic month is always counted out in correspondence with a whole-day rate (29 days) then the difference (when counted apart as a day rate) inherently equals 105 additional days in every cycle of 16 tropical years. (This respective rate of additional days is also exactly equal to 735 days in a cycle of 112 years).
The indicated correspondence between the spin of the Earth and the synodic revolution of the Moon is then remarkable in the regard that 105 days in 16 years . . . or 735 days in 112 years . . . is quite perfectly equal to the rate of 0.53059 days per lunar month. In essence, a 30th day in the Moon's synodic obit can be counted at a rate that is exactly equal to 105 days in a cycle that is precisely equal to 16 years.
________________________________________ A PRECISE DAY-TO-YEAR CORRESPONDENCE BASED UPON THE SYNODIC MONTH ________________________________________ Cycle Number of Synodic Month Days Tropical Months at in Excess Years 29 Days of 29 Days ----- -------- --------- ---------- 1 16 197.89225 105 2 16 197.89225 105 3 16 197.89225 105 4 16 197.89225 105 5 16 197.89225 105 6 16 197.89225 105 7 16 197.89225 105 ---------------------------------------- Totals: 112 Y. 40172.1277 D. 735 D ________________________________________ Total Days for Model = 40907.1277 days Length of 112 Years = 40907.1253 days Month Rate for Model = 29.5306 days Synodic Month Rate = 29.5306 days
Thus, the rate of the lunar-month in excess of 29 days (or 105 days in 16 tropical years) can be recognized to inherently bound with the epoch of each 16th year to within an average difference of only 30 seconds. (This is a difference of less than 2 seconds per year!)
The cited rate of 105 days can then almost perfectly be scribed relative to the rate of 16 tropical years; however, because the spin of the Earth appears to be slowing with time then this respective solar-day count can be predicted to have been absolutely perfect in the relatively recent past.
The cited rate of lunar-month days in excess of 29 days is then of considerable significance in the regard that the number of days in each synodic revolution CAN systematically be scribed relative to always 29 days (as a rate of whole days). Of further significance is that the stated rate of days in excess of 29 days can also be recognized to exactly (perfectly!) interface with the rate of the tropical year.
Because the required secondary rate of days is all but perfectly equal to 105 days in every time segment of 16 tropical years then it is clear that the ancients could very well have 'day counted' the synodic return within the context of this respective time cycle.
Note that if the period of the Moon cycled at a rate exactly equal to 29.5 days per synodic month then a 30th day could simply be intercalated every alternate month. However, the actual synodic revolution in 29.53059 days is 44 minutes and 3 seconds slower than 29.50000 days. This respective difference--if prorated on a straight-line basis--would mandate that an additional day always be intercalated every 55.65614 days (on the average). [Note that each tropical year inherently contains 12.368267 lunar months. This number of months when multiplied by an excess over 29 days of 0.53059 days per month is equal to 6.56248 additional days per tropical year--on the average. (This rate is also equal to 1 additional day every 55.65614 days . . . or is equal to 104.99965 additional days every 16 tropical years).].
________________________________
Of related significance is that early-written literature indicates that primal priest-astronomers once used axioms and time formulas to effectively measure and meter the lunar and solar periods. (Certain of these early used axioms/formulas are so very accurate that even a contemporary/modern astronomer would find them to be of use). For additional information concerning the early use of axioms and time formulas, refer to the following online publications:
For additional information about functional time design, refer to the following online publications:
Please feel free to download and distribute the current article, or any of the articles and booklets listed above. (Note that the published material is subject to constant revision. Be advised that corrections, amendments, and new interpretations are frequently made.)
Copyright © 2007-2021 A-Quest-For-Creation-Answers