Time Cycles of Ancient Astronomy

_______________________
Adages and axioms
Early astrologers and astronomers can be recited to have tracked specific time cycles in association with the orbital returns (Sun and Moon).
Axioms and certain adages appear to have also been followed--in association with a scribing method--to measure and meter out the celestial circuits.
Perhaps the most ancient collection of once-used axioms and time formulas can be recognized from a manuscript attributed to Enoch (one of the Bible patriarchs). It is here significant an entire section of this early-written literature focuses upon "the revolutions of the heavenly luminaries". (The section detailing the "revolutions of the heavenly luminaries" is known as Enoch's astronomical book).
Among the most intriguing of the lunar-solar relationships detailed in Enoch's astronomical book is the description of a whole-number of days (a rate of lag) between 8 lunar years (or 96 synodic months) and 8 solar years.
To be more specific, the noted lag-rate relationship is unusual in the regard that a whole number of day units does appear to exist between the boundary of 96 synodic months and 8 solar years.
Note that the number of days in 8 solar years are 2921.93752 days; while the actual days in 8 lunar years (or 96 lunar months) are 2834.93664 days. The day difference in 8 solar years and the corresponding lag in lunar periods is then a whole-day difference of 87 days.
2921.93752 solar days minus 2834.93664 lunar days ----------------------------- equals 87.00088 days of lag
The description of this lag-relationship is entirely valid and indicates that even very early astronomers may have been effective in tracking various Earth-Moon cycles.
When describing this particular lag-relationship, the cited Enoch literature shows a whole-day difference of 80 days (not the correct whole-day difference of 87 days). The difference of 7 days--as is indicated in what has survived from the Enoch literature--is consequently somewhat of a mystery.
In the light of modern astronomy, the cited cycle of 8 solar years and lunar-lag relationship is of special interest in regard that a small amount of lag difference can currently be observed between the rate of the synodic month (of 29.53059 days) and a whole-day count of 29 days.
The lag difference between the two rates averages out to be a little over half a day--or 0.53059 days. (Note that 29.53059 days minus 29 days is equal to 0.53059 days).
Based upon this indicated half-day count difference, it follows that if the rate of the synodic month is always counted out in correspondence with a whole-day rate of 29 days, the cited difference of the half-day rate (0.53059 days per lunar month) would eventually accumulate or accrue to the sum of exactly 105 half days in every cycle of 8 solar years.
This inherent correspondence reveals that the rate of 105 half days in 8 years is all but perfectly equal to the rate of 0.53059 days per lunar month). Essentially, 0.53059 days per lunar month--if extended for the number of months in 8 years or for 98.94613 lunar months--is precisely equal to the length of 105 half days.
This ultimately means that if each synodic period is systematically scribed relative to always 29 days, and if 105 half days are additionally scribed relative to the passage of 8 years, the boundary of every 105th half day will inherently correspond with the boundary of 8 solar years--on the average.
Thus, the number of lag days in each synodic period of the Moon CAN be used to precisely scribe the limits of a cycle of 8 solar years (in average time). A scribe of 29 days per lunar month and the additional scribe of 105 half days has an 8-year average that equals 5843.87555 half days. (This 8-year average quite perfectly correspondends with the limits of 8 solar years--which is equivalent to 5843.87504 half days).
The cited scribe of lunar periods and half-day cycles (on the average) can be recognized as a very precise method of determining the limits of 8 solar years. In this modern era, the cited method of scribing half days averages out to be only 0.00051 more days than an actual cycle of 8 solar years. The average result of the cited scribe is almost perfect. (Due to the tiny rate by which the spin of the Earth appears to be slowing down, the cited scribe of 8 years can be predicted to have at one time been absolutely perfect. The time when a perfect scribe was possible can be predicted at about 20 centuries ago.)
Track of a 30-day cycle
The effectiveness of ancient astronomers in measuring and metering the orbital returns can further be recognized from those records that indicate the track of a 30-day cycle.
The primal count of a cycle of 30 days can especially be recited from portions of the previously cited astronomical book of Enoch.
For pertinent information about a set of adages and axions embeded within Enoch's astronomical book, refer to an online document entitled:
The following quote illustrates that the count of a 30-day month may have been in use from a very early time in the ancient Middle East:
"The old Babylonian year consisted of 360 days--twelve months of thirty days each... The Assyrian year contained 360 days; a decade was made up of 3,600 days. Assyrian documents reveal a thirty-day month... Anciently, the Persian year also had 360 days of twelve months containing thirty days each. The Egyptian year was 360 days in length... The Mayan year originally consisted of 360 days... In South America, in ancient times, the year consisted of 360 days with twelve months. The same was true in China--360 days with twelve months... Plutarch wrote the Roman year was [originally] 360 days. Various Latin authors record the month as being thirty days in length... The Hindu year was made up of twelve months of thirty days each... All the historical computations found in Hindu history used a 360-day year with months of thirty days each... (Worlds in Collision, Velikovsky, 124, 331-341).
It seems clear that a 30-day cycle may have been reckoned throughout the ancient world.
"During the reign of Romulus... they only kept to one rule that the whole course of the year contained three hundred and sixty days". (Lives, The Life of Numa, by Plutarch, translated by John Dryden).
The reckoning of a 30-day month would, of course, have required periodic intercalation--where, amid a count of 30-day months, intercalation would specifically have been required at the rate of 5 days per solar year.
In some early-used, time-tracking systems it is of interest that intercalation appears to have been deferred beyond the limits of only a single year.
According to an early Assyrian method of time tracking, calendar intercalation was determined on the basis of a running '10 count':
"The year attested in Kultepe texts... every three years [required] the insertion of 15... days called shapattum... Throughout the [3 years] Assyrians counted ten-day periods ... For three years, these [counts] ran congruently with the months and the years. Then, after the insertion of a 15-day shapattum period, they overlapped from one month into the next, returning to congruency with the months after the next shapattum...." (From Britannica, 1972, Calendar, Babylonian And Assyrian Calendars).
In the Kultepe calendar, it is significant that a 3-year cycle was reckoned and also a half-month cycle appears to have then been intercalated. Furthermore, a repetitive count of '10' was used in correspondence with each calendar month of always 30 days. Of additional interest is that the cited half-month interval (shapattum) would inherently have renewed in association with the running count of the '10 days'.
The half month would have either been overlapped, or renewed in coincidence with the unbroken count of '10'. Prior to a shapattum, the count of 10 would hypothetically have progressed across each month of 30 days in correspondence with month-day 10... month-day 20... month-day 30... etc. After the first shapattum (or after the insertion of the half month) the count of 10 would then have progressed across each of the 30-day months in correspondence with month-day 5... month-day 15... month-day 25... etc. After reckoning the second shapattum, the count of 10 would have then progressed again in correspondence with month-day 10... month-day 20... month-day 30... (or the same as before the first shapattum).
A plausible interpretion of the cited count of 10 is that this unbroken count was performed to define "stations" of the Sun and Moon. Of significance here is that--by accounting for stations of the Sun and Moon--early priest-astronomers would inherently have been capable of performing an accurate (even perfect) measure of each passing tropical year.
For pertinent information about a time track of lunar and solar stations, refer to the following online publications:
Zodiac calendars
Historic literature additionally indicates the ancients held knowledge of the tropical zodiac (12 annual divisions).
Of significance here is that a segment of early astronomers appear to have interpreted certain lunar days in association with the circle of the zodiac.
- "Hermes playing at draughts with the Moon, won from her the seventieth part of each of her periods of illumination, and from all the winnings he composed five days, and intercalated them as an addition to the 360 days." (Isis and Osiris, Plutarch, translated by F.C. Babbit).
- "[The Egyptian gods played] dice with the Moon and won five days a year. Because these days were outside the [solar] calendar, Re's decree did not apply." (Time Incorporated, 1966. p. 69, Samuel A. Goudsmit).
An intruiging interpretation of how counts of both lunar and solar days can be used to track 360 degrees of time in each annual circle can be derived from certain artifacts of early stick calendars.
For example, a pictorial calendar from prior to the time of Titus (A.D. 79-81) indicates a repetitious day-by-day count of certain time cycles. Furthermore, a charting of the 12 signs of the zodiac is indicated from the appearance of the cited pictorial calendar (Attilio Degrassi, Inscriptions Italiae, 1963, XIII, pp. 308-309, refer to plate 56).
Some historic artifacts are likewise clear in indicating that specific day cycles were once tracked to augment charting the circle of the zodiac as well as the 7 planetary domains.
In addition to the planetary domains, early zodiac calendars are found to have also sometimes contained two vertically positioned columns with 30 numbered holes.
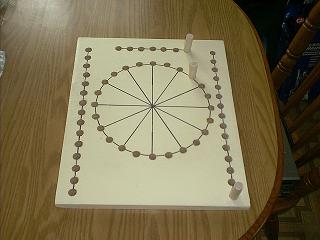
The shown photograph is of a replica largely based upon a stick calendar from Saint Felicity oratory in Rome.
It is obvious from the cited artifacts that time cycles were sometimes tracked by the moving of a peg relative to a predetermined arrangement of markers or bored holes. (Updating of the location of a peg to another marker or hole would have been required on a daily basis).
In exploring more of how the ancients would have used the above shown calendar, a knob or a stick would have been moved in correspondence with the top row comprised of 7 holes. Another knob or stick would have been moved in correspondence with the two columns consisting of 30 numbered holes. Yet a third knob would have been periodically moved around the center circle of holes to track the circle of the zodiac.
From the 30 numbered holes shown on certain among the early used zodiac-calendars, it seems certain that a 30-day cycle was reckoned in association with 12 divisions in each annual cycle.
The cited row of 7 holes that appears on some historic artifacts does almost surely indicate that a planetary cycle of 7 days was simultaneously being time tracked--as is further shown below.
For a technical discussion concerning how the cited stick calendar could have been used in an effective track of the tropical year, refer to the online publications previously listed.
The week cycle
The cited artifact of a stick calendar indicates that a week cycle of 7 days may have been known among the Romans as early as the first century.
It is--however--not clear from the historical record that first-century Romans would have subdivided time in units of the 7-day cycle.
The Roman practice of counting the 7 planetary days probably began to grow popular about the beginning part of the second century.
The usage of the week among the Romans in the third century is attested to by Dio Cassius (c. 200-220 CE) who wrote: "The dedication of the days to the seven stars which are called planets was established by Egyptians, and it spread also to all men not so very long ago... [This dedication now] prevails everywhere..." (Dio Cassius, Historia 37,18).
The interpretation of a week cycle of 7 planetary days--as it would have been understood by Romans of the third century--appears to have originated among earlier Babylonian and Egyptian astronomers. (More primal Babylonian and Egyptian priests appear to have reckoned a running cycle of 7 hours in association with 7 planetary gods). The Romans came to ultimately believe that the 7 planetary gods were to be honored in a specific order or sequence--as follows: 1. Saturn; 2. Sun; 3. Moon; 4. Mars; 5. Mercury; 6. Jupiter; 7. Venus.
While the week cycle did not grow to become popular among the Romans until the second century, the use of a week cycle was already popular in regions of the Middle East from more ancient times. It is here of interest that astronomers in this respective region appear to have tracked a cycle of 7 running days all throughout the Second-Temple Era. Furthermore, a running count of the week--a king's cycle--can be recited to have been in use from prior to the sixth century BC.
For pertinent information concerning the early time track of "weeks of days" and "weeks of years", refer to the following online publications:
The indicated Egyptian track of the annual circle in time segments of 10 degrees (36 segments per year) points to the possibility that Egyptian astronomers may have been knowledgeable of a 70-day cycle--and from very ancient times. The Egyptian reverence for a cycle of 70 days can seemingly be recited from the Bible book of Genesis (refer to 50:3). It here becomes significant that--if the rate of one day is routinely scribed in association with a cycle of 70 days--the residual days inherently become equal to 360 days (360.0245 days) on an annual basis. This accurate annual scribe means that an ancient astronomer could have effectively measured and metered out each and every degree of the annual circle through the simple scribe of a 70-day cycle.
Lunar and solar cycles
In summary to the above, it is clear that--through the reckoning of cross-referenced lunar and solar cycles--it would have been possible for early astronomers to very effectively measure and meter each passing tropical year.
It here seems to be of significance that in addition to an accounting of solar days, the reckoning of special lunar days may have also been performed.
For additional information concerning the combinational time track of both lunar and solar cycles, refer to the following online publications:
____________________________________
Appendix A
A Scribe of 20 Days
A study of cosmological interpretations embraced by ancient cultures of South America reveals the unilateral adherence to a time track of 20 days.
Of significance is that--even in this modern era--a cycle of 20 days continues to be tracked among some of the Indian groups resident in this region. According to what must be a centuries old tradition, each day of a never-ending cycle of 20 days is interpreted to correspond to the order of 10 fingers and 10 toes. Consequently, this still adhered to cycle seems to mirror a somewhat similar count of 10 days (a count once popular in the ancient Middle East).
Data pertaining to the ancient Aztec Calendar indicates that each day of a running 20-day cycle was named in correspondence with a specific god or deity. (The indicated 20 names for the days of this cycle appear to have differed between the various Indian cultures).
This peculiar running count of 20 days can be recognized to have been integral among cultures in South America in the definition of a number of early-used time cycles. For example, a significant time cycle once tracked was a week-like cycle of 13 days. This respective 13-day week--reckoned in association with the cited cycle of 20 days--was used to define a long cycle of 260 days. (Note that 260 days inherently contains 13 cycles of 20 days. Also 260 days inherently contains 20 cycles of 13 days. Essentially, both cycles appear to have been interpreted to conjoin and to define a cycle of 260 days).
For pertinent information, refer an article published in the May 2004 online edition of the Wikipedia Encyclopedia:
It is unusual that almost all of the cosmological interpretations embraced by the Indians of South America appear to have been rooted in repeating counts of the two cited time cycles:
- A month-like cycle of 20 days
- A week-like cycle of 13 days
Of further significance is that not only were cycles of 13 days repeatedly counted but so were cycles of 13 years repeatedly counted. It is manifest that after every 4 cycles of 13 years (or after 52 years) the years were perceived to become "bundled up" or "collected". Essentially, the early Indians appear to have attributed some certain significance to the limits of a "calendar round"--where each round of the calendar was interpreted to be equivalent to a cycle of 52 years.
This concept of the years becoming "bundled up" in association with a "calendar round" of 52 years can possibly be recognized in the root definition of 20-running-days. It seems to here be of significance that the cited cycle of 20 days was perceived by the Mesoamericans to pass sequentially from the 1st day up to the 19th day. The cycle of 20 days was renewed in association with a specially numbered day (day zero). The cyclical count, beginning with day zero and counted up to the 19th day, tends to indicate that the early Indians interpreted a 20-day cycle in which 19 of the days were specially numbered or scribed. (Throughout each tally of 20 days, day zero may have been accounted for apart from the other 19 days that comprised the cycle).
It here seems to be signficant that the routine scribe of 19 days out of every 20 days can be stated in terms of a percent. Essentially, the reoccurrence of an uncounted day (or day zero) inherently occupies 5 percent of the time stream.
Note that 1 day in 20 days is equivalent to 5 percent of time.
It then becomes a given conclusion that the renewal-day rate (5 percent of the time stream) is equivalent to a rate of 18.26211 days on an annual basis.
Note that 5 percent of each solar year (or 5 percent of 365.24219 days) is inherently equal to an annual rate of 18.26211 days.
From the annual rates of cited scribed days and unscribed days, it can be recognized that each solar year can effectively be measured and metered out in association with 347 scribed days.
The rate of the solar year (365.24219 days) minus the cited annual rate of unscribed days (18.26211 days per year) is inherently equal to residual number of scribed days (or 346.98008 days per year).
A scribe of always 347 days in correspondence with the passing of each solar year would ultimately require the count or scribe of day zero one time in every "calendar round" of 52 years.
Note that 347 scribed days per year plus 18.26211 unscribed days per year for 52 years inherently achieves an average calendar count of 18993.62969 days. If the years are "collected" or "bundled up" through an exception for the scribe of day zero at the frequency of every 52 years then the number of calendar days in each calendar round becomes inherently reduced by the count of 1 day. Thus, it is possible to interpret from the cited 20 day scribe that early Mesoamericans might have once reckoned each "calendar round" of 52 years to within the limits of 18992.62969 days (on the average). (This determination of the calendar round rather closely compares with the length of 52 solar years--which is 18992.59388 days).
In any case, it is remarkable that a tally of unscribed days (the rate of 1 day in 20 days) can be used to effectively measure and meter out the rate of each passing solar year. (If 19 days in a cycle of 20 days are routinely counted, or scribed, than the length of each year can always be correlated to a scribe of 347 days).
It here seems to be additionally significant that were 5 percent of each solar year (or 18.26211 unscribed days) accounted for separately from the remaining 95 percent of the solar year (or 347 scribed days) then the very best long cycle by which the years should be "bundled up" or "collected" would be in a jubilee cycle of 50 years (not in a "calendar round" of 52 years).
This rather perfect jubilee correspondence is easy to recognize from the cited average number of unscribed days per solar year (18.26211 days). This rate... when combined with the cited number of scribed days per solar year (a rate of 347 days per year)... inherently achieves a rate of 18263.10548 days per jubilee cycle of 50 years. A given conclusion from the combination of rates then is that if all 20 days of the eternally revolving 20-day cycle were counted out one time in each jubilee cycle then a jubilee rate of 18262.10548 scribed and unscribed days is the inherent result. This respective rate of days can then be recognized to compare almost perfectly with the actual length of 50 solar years (which length is equal to 18262.10950 solar days).
The cited scribe of 20-day cycles across a long cycle of 50 years then appears to be a very effective method of determining the limits of each and every year of a jubilee cycle (50 years). In this modern era, the cited scribing or accounting method (347 scribed days per year) can be used to ultimately determine the average reoccurrence of each passing solar year to within the limits of 7 seconds per year (on the average).
It here seems pertinent to point out, in the near future, the reoccurrence of each year of a 50-year cycle should become perfectly aligned with the cited result of scribing 20-day cycles. (Due to the slowing spin of the Earth, it can be predicted that an exact average alignment will occur about 19 centuries from now). For pertinent information concerning the slowing spin of the Earth, refer to the online publication:
The calendar adhered to by early Mesoamericans is of additional interest in the regard that their week plan of 13 days appears to have been used to determine a long cycle of 364 days. (Note that 28 weeks of 13 days per week is equal to 364 days). The indicated running count of the week (364 days per year) can be recognized to be similar to a weeks calendar once popular in the ancient Middle East. In the region of the Middle East, it is manifest that a week cycle of 7 days was cycled 52 times so as to achieve the same calendar count (364 days).
As in early Mesoamerica, the Middle-Eastern count of a week cycle was continuous. Essentially, the week was counted on-and-on as an unbroken cycle.
In the Middle East, the running week cycle (7 days) was tracked so as to ultimately define a long cycle of 7 years. The long count of 7 years was then used to ultimately determine/define great time cycles (or time cycles comprised of "weeks-of-years").
Of possible significance is that the week cycle of Mesoamerica appears to have been counted so as to achieve a long cycle of 364 years. Some chronographers account that the cited cycle of 364 years did renew in correspondence with the year 1091 CE. If the anciently reckoned cycle of 364 years did renew in the year 1091 CE, it then becomes of special interest that the cycle of 7 years (a cycle once tracked in the region of Middle East) did also renew in correspondence with this very same year (1091 CE). As such, the "weeks-of-years" reckoned throughout ancient Mesoamerica and the "weeks-of-years" reckoned throughout the ancient Middle East can be recognized as synchronized time cycles. Essentially, the year 1091 CE would inherently have coincided with a 49th year as an extension of the well-documented cycle of 7 years once celebrated in the region of Judea. For pertinent information of the celebration of a weeks calendar in the ancient Middle East, refer to the online document entitled:
____________________________________
Appendix B
A Scribe of 40 Days
A time track of 40 days can be recognized from the book of Genesis--and from some other books of the Pentateuch.
Of possible significance is that the epoch of each passing solar year can be determined to within the limits of only 2 seconds (in average time) by keeping track of this respective cycle.
For pertinent information about a calendar count of "40 days and 40 nights", refer to the following online publication:
____________________________________
Copyright © 2002-2021
A-Quest-for-Creation-Answers
Please feel free to download and distribute--but not sell--the articles and booklets listed above. (Note that the published material is subject to constant revision. Be advised that corrections, amendments, and new interpretations are frequently made.)
_________________________
RELATED READING
Priest-astronomers in the ancient past appear to have been effective (even perfect) in measuring and metering out the apparent lunar and solar orbits.
_________________________
Please feel free to download and distribute--but not sell--the articles and booklets listed above. (Note that the published material is subject to constant revision. Be advised that corrections, amendments, and new interpretations are frequently made.)