<Return to Home Page>
Is There a Case for Created Time?

We, as residents of Planet Earth, can expect a new day to arrive each and every 24 hours, a new lunar month to come after 29.5306 days, and a new year to return in 365.2422 days . . . but what are we missing here?
By: James D. Dwyer
Last revised: August 2016 (+9)
Copyright © 2007-2021 James D. Dwyer
Email: quest@creation-answers.com
Reference: www.design-of-time.com
You may freely copy or distribute this material.
(Not to be sold).
_____________________________
Introduction
From a perspective that the Earth-Moon-Sun might represent the work of Divine Creation, the orbital rates are quite puzzling. A most primary concern here is that the lunar month (of 29.5306 days) cannot be divided into an even number of 24-hour units. Furthermore, the tropical year (of 365.2422 days) turns over in pace with the fractional portion of a day.
In an effort to demonstrate that the spin and orbital rates CAN ALL be interpreted within the context of intelligent time design, the current research looks at certain passages from the Bible (and related texts) for some help. Of significance here is that an effective time model of the Earth-Moon system is easy to document (yes, thanks to information contained in ancient literature).
Subsequently presented paragraphs and pages have all the detail; and as stated above, the presented focus is upon a luni-solar system that was penned and described by a segment of earlier astronomers. (It should here be noted that the current documentation is predicated upon modern spin/orbital rates).
_____________________________
Finding lunisolar time design
From a time-design perspective, the Earth-Moon system is enigmatic in that the various spin and orbital rates seem to be unrelated. In essence, when compared all together, rates of days, lunar months, and years seem to point to a system that is out of sync and misaligned.
But let's assume that the Earth and Moon might possibly mirror some kind of plan from a higher level . . . that of the Divine. Of interest here is there are a few conjunctions to wonder about--such as when two cycles comprised of the rates of either solar days, synodic months, or solar years have the same length. In essence, certain time structures ARE uniquely defined when the rates of either days, months, or years periodically conjoin or interface together.
One such time structure (a perfect structure) is defined by an ongoing progression of 7 lunar months.
A cycle of 7 synodic months--when cycled 7 times--can be recognized to revolve into rather perfect alignment with the same spin-phase of the rotating Earth. Essentially, a rate of whole days (1447 solar days) appears to precisely align with or come into conjunction with 7 sets of 7 lunar months (or 49 synodic months).
Note that 1447 solar days divided by the rate of the synodic-month cycle, or 29.53059 days, is equal to 49.0000 lunar months.
The following diagram is presented to more fully illustrate that a cycle of 7 lunar months (cycled 7 times) very closely interfaces with the rate of the rotation of the Earth:
____________________________________ THE INTERFACE OF 49 SYNODIC MONTHS ____________________________________ Number of Number of Months * Earth's Rotations --------------------------- ------- 1 2 3 4 5 6 7 206.71 8 9 10 11 12 13 14 413.43 15 16 17 18 19 20 21 620.14 22 23 24 25 26 27 28 826.86 29 30 31 32 33 34 35 1033.57 36 37 38 39 40 41 42 1240.28 43 44 45 46 47 48 49 1447.00 --------------------------- ------- * - A span of 49 lunar months is equal to 1447 days.
It here seems possible to interpret that the cited Earth-Moon interface (a precise interface at 49 Moons) could represent interrelated time design. (Note that the modern rate of 49 synodic months happens to almost be perfect relative to the same spin-phase of Earth's rotation).
Not too many well defined time structures (such as the cited time structure comprised of 7 lunar months) can be interpreted from the current spin-orbital rates. Nevertheless, it remains to be significant that the Earth-Moon inherently does define certain time structures that appear to be close to perfect. (Because certain time structures can actually be interpreted from the past and present spin-orbital rates then it becomes more plausible to at least suspect that the Earth-Moon may represent Divine planning).
Here, it seems pertinent to note that a single conjunction cycle is not fully convincing evidence of a system that is functionally related. This is because any two time cycles progressing at different rates eventually come into conjunction. This means that before any fully satisfactory interpretation of an intelligently ordered Earth-Moon can be arrived at then more of a characteristic of functional interrelatedness needs to be recognized from out of the spin-orbital rates. Fortunately, this kind of interpretation can actually be made, and--remarkably--the rates of solar days, synodic months, and solar years can rather convincingly be demonstrated to comprise a time-tracking system that is functionally related. The remainder of this document will then attempt to make clear that the Earth-Moon system can (and probably should) be interpreted as a system that is fully interrelated.
The 7-year cycle
Another good example of the Earth and the Moon as an interrelated system can be recited in a time cycle of seven years. (This respective cycle has been used to track time with for literally thousands of years and consequently it is somewhat remarkable that this time circuit is inherently illustrative of an intelligently ordered system).
Significant in documenting a relationship between the synodic Moon and a cycle of 7 years is that each lunar quarter is equal in length to 7.38265 days (on the average).
An Earth-Moon model comprised of '7 sets' is possible using the somewhat peculiar unit of the lunar quarter. It is here significant that the synodic month defines a unique quarter-phase cycle as it passes through the following four specific phases: (1) New phase (when the Moon is fully dark); (2) First-quarter phase (when the Moon is one-half illuminated); (3) Full phase (when the Moon is wholly illuminated); and (4) Last-quarter phase (when the Moon is again one-half illuminated--but on the alternate side.)
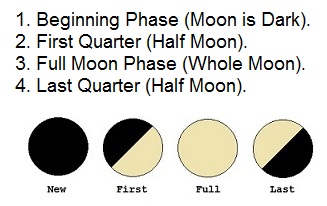
For the purposes of presenting a clear analysis, the unit of the lunar quarter will hereafter be referred to as the lunar week. It is here significant that the unit of the lunar week (the lunar quarter) is equal in length to 7.38265 days (on the average). The lunar week is consequently a bit longer (or slower) than an ordinary week of 7.00000 days.
The following diagram is presented to show that the unit of the lunar week (in 7 sets) can be used in the definition of a series of 7 years (in 7 sets):
______________________________________ 7 sets of 7 years can be defined by counting 7 sets of 7 lunar weeks ______________________________________ 7-Yr No. Number of At Each Seg Yrs Lunar Weeks 7th Year ---- --- ----------------- -------- 1. 7 7 times 7 times 7 + 1 week 2. 7 7 times 7 times 7 + 1 week 3. 7 7 times 7 times 7 + 1 week 4. 7 7 times 7 times 7 + 1 week 5. 7 7 times 7 times 7 + 1 week 6. 7 7 times 7 times 7 + 1 week 7. 7 7 times 7 times 7 + 1 week ---- --- ----------------- -------- 50th = 1 yr 7 times 7 ______________________________________ Note that a leap week (a 3-year rate) is required to keep the depicted 7 sets into alighment with 7 sets of years.
The diagram shows that a lunisolar calendar is feasible. The calendar shown requires the leap of a lunar week each 3rd year (a perpetual rate).
The table shown above outlines the existence of a cross-reference between a grid of 7-year cycles and a grid of lunar weeks. Because of this cross-reference, an effective calendar of lunar weeks is possible (as is further shown below).
Note that--throughout 7 sets of 7 years--each calendar segment of 7 years can be equated to a fixed lunar-week count of 7 times 7 times 7. (The fiftieth year--as shown--is uniquely defined and delimited by a lunar week count of 7 times 7).
It is noteworthy that a calendar count of 7 times 7 years (or the jubilee cycle) is explicitly outlined within biblical and associated ancient texts. (For additional information of this 7-year count, refer to the online publication entitled:
Because a 7-year model (as diagrammed above) can be interpreted from out of the spin-orbital rates, it becomes plausible to at least suspect that the Earth-Moon may represent a fully interrelated system.
A degree of interrelatedness between the rate of the synodic month and the rate of the solar year is obvious in the regard that a common denominator (the lunar quarter) can be extracted from both rates. A systems view of the Earth-Moon--expressed in units of lunar weeks--initially might seem to be a bit on the odd side. Nevertheless--and amazingly so--a fixed count of weeks nicely interfaces with the rate of the solar year (as is further documented below).
The annual interface with 7 lunar weeks
The lunar-week (or the lunar quarter) can be recognized to interface with a time grid comprised of 7-year cycles--as is shown in the previously presented section.
Of related signficance is that a calendar count of the lunar week can likewise be recognized to correspond with the rate of each passing solar year (on the average).
The following diagram illustrates that a time grid of lunar weeks can very closely be correlated or cross-referenced to a time grid of solar years:
---------------------------------------- A JUBILEE CALENDAR OF LUNAR WEEKS ----------------------- -------------- Year 1: 49 weeks Year 8: 49 weeks Year 2: 49 weeks Year 9: 49 weeks Year 3: 49 weeks Year 10: 49 weeks Year 4: 49 weeks Year 11: 49 weeks Year 5: 49 weeks Year 12: 49 weeks Year 6: 49 weeks Year 13: 49 weeks Year 7: 49 weeks Year 14: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 15: 49 weeks Year 22: 49 weeks Year 16: 49 weeks Year 23: 49 weeks Year 17: 49 weeks Year 24: 49 weeks Year 18: 49 weeks Year 25: 49 weeks Year 19: 49 weeks Year 26: 49 weeks Year 20: 49 weeks Year 27: 49 weeks Year 21: 49 weeks Year 28: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 29: 49 weeks Year 36: 49 weeks Year 30: 49 weeks Year 37: 49 weeks Year 31: 49 weeks Year 38: 49 weeks Year 32: 49 weeks Year 39: 49 weeks Year 33: 49 weeks Year 40: 49 weeks Year 34: 49 weeks Year 41: 49 weeks Year 35: 49 weeks Year 42: 49 weeks At 7th Year: 1 week At 7th Year: 1 week ------------------- ------------------ Year 43: 49 weeks Year 44: 49 weeks Year 45: 49 weeks Year 46: 49 weeks Year 47: 49 weeks Year 48: 49 weeks Year 49: 49 weeks At 7th Year: 1 week ------------------- Year 50: 49 weeks
It should be clear that when the count of one lunar week each and every 3rd year (the X1 rate) is subtracted from out of a streaming count of lunar weeks (or lunar quarters), a jubilee calendar comprised of lunar weeks is the inherent result. Essentially, a streaming count of lunar weeks (or lunar quarters) can be used to precisely define each year of a 50-year cycle.
Note that each calendar year -- on the average -- is equal to 365.2442 days and this length compares very closely with the rate of the solar circle or year-- which completes in 365.2422 days.
Thus, it becomes of considerable significance to a study of interrelated time design that an effective annual calendar is the inherent result of counting 7 lunar weeks.
The above shown calendar of lunar weeks would inherently remain accurate relative to the pace of the tropical year over many centuries of time. The time difference between the respective 49-week calendar and the length of the solar year (which turns every 365.2422 days) would eventually become a factor if enough time were to pass by. To be specific, assume that a new phase of the Moon was observed (as the first day of the calendar) at say 7 days prior to the day of the vernal equinox. From this origin and alignment, the first day of the calendar would inherently shift (on average) from year to year so that after 3600 years the first calendar day would arrive in alignment with the equinox, and after 7200 years the first calendar day would come 7 days after the equinox. What turns out to be amazing is that a cycle of 7 lunar weeks can also be interpreted within the context of calendars that are even more accurate. For more incredible detail about the calendrical significance of this respective time cycle, refer to the following online publications:
An interpretation of time stations
An ancient Biblical patriarch named Enoch is reputed to have left record of the year becoming "complete according to the station of the Moon, and the station of the Sun". This notation of 'time stations' seems significant in the regard that the length of each passing solar year can very effectively (almost perfectly) be measured and metered by simply counting solar days. In essence, the length of the solar year (365.24219 days) can almost EXACTLY be correlated to a fixed number of annual days. (This axiom is valid in the context of additionally counting days positioned eternally at Sun and Moon stations).
To be more specific about the definition of time stations, a day count of the tropical year is possible within the context of tracking only the following two time cycles:
- A cycle defined by the Moon.
- A cycle defined by the Sun.
The first of the two cited time cycles that must always be accounted for is equal to 7 lunar weeks.
------------------------------------ Moon Cycle of 7 Lunar Weeks ------------------------------------ Lunar quarter 1 (lunar week) Lunar quarter 2 (lunar week) Lunar quarter 3 (lunar week) Lunar quarter 4 (lunar week) Lunar quarter 5 (lunar week) Lunar quarter 6 (lunar week) Lunar quarter 7 (lunar week) ------------------------------------
The revolution of a time span equal to 7 lunar weeks must eternally be time tracked -- as follows: 7 lunar weeks . . . 7 lunar weeks . . . 7 lunar weeks . . . (and so on and on).
The second of the two time cycles that must always be tracked is equal to the span of time occupied by 30 solar days. The revolution of a time span equal to 30 solar days must eternally be time tracked -- as follows: 30 days . . . 30 days . . . 30 days . . . (and so on and on).
Of significance here is that one of the best possible day-count models that can account for each passing tropical year requires only a running count of the two stated cycles: of the Moon, and of the Sun. (Note that amid all the days that occupy the time stream, each of the 30th days must be separately accounted for, and each day at the 7th lunar week must likewise be separately accounted for).
The following diagram more specifically illustrates the feasibility of 'day counting' a span of time equal to the solar year. The current model only requires an accounting of the Moon and Sun cycles--as follows:
------------------------------------- EARTH'S ROTATION CAN BE CORRELATED TO THE ANNUAL QUARTERS ------------------------------------- Annual Corresponding Division Day Counts -------- ----------------------- Quarter 1 1 + 28 + 29 + 28 Quarter 2 1 + 29 + 28 + 29 Quarter 3 1 + 28 + 29 + 28 Quarter 4 1 + 29 + 28 + 29 ------------------------------------- In order to remain in pace with the seasonal turns, the shown diagram of days requires the addition of Sun and Moon stations--as follows: 1. Every 30th day (+ 1 day). 2. Every 7 lunar weeks (+ 1 day).
The above diagram shows that--in pace with unique separately counted days or time stations--each passing annual-quarter division can easily and effectively be metered.
Please take note here that an intercalation rate equal to 1 day per Sun Cycle and 1 day per Moon Cycle is equal to 19.24232 days per year. This then means that from year to year the seasonal turns can effectively be metered out in correspondence with a fixed count of days. Note that a calendar count of 346 days with intercalated days achieves an average solar-year rate of 365.24232 days.
Thus the average result of tracking days within the context of Sun and Moon cycles (in this modern era) is proven to be perfect from year-to-year within a difference of only 11 seconds! (The annual result of tracking celestial time stations can be recognized as fully or absolutely perfect only centuries before).
Refer to the following online publications for more complete information concerning the significance of tracking celestial time stations:
A two-count system for time tracking
The time-tracking system subsequently shown requires the day count of a primary cycle as well as the day count of a secondary cycle.
The currently described day-count system is significant in that the turn of each passing tropical year can very accurately be determined. It should here be mentioned that a double-count system also has significance relative to yet a third time cycle which revolves every 7 lunar weeks.
To be a bit more specific about a system that is effective for measuring and metering the cycle of the tropical year, the count of primarly and secondary time wheels are required. In association with counting the cited two cycles, an exclusive day count can then be equated to the revolution of the tropical year. In essence, an annual calendar can be derived from out of the revolution of two time cycles.
In terms of accuracy, a two-count system can achieve an annual calendar that paces the cycle of the tropical year to within an average difference of only 2 seconds. This means that the cited primary and secondary cycles can remain of fixed length, and no additional insertion or deletion of days is ever required, even across a time run of many thousands of years. The accounting of only two short cycles can thus achieve what is probably the most accurate calendar that can be derived from out of the spin and orbital phenomenon.
The subsequently presented time-tracking system is predicated upon a solar day of 24 hours (or also 86400 seconds) and a tropical year of 365.24219 days (or also 365 days, 5 hours, 48 minutes, and 45 seconds).
The effectiveness of a two-count system can perhaps more quickly be grasped by first forming a focus upon a continuous time track of 40 as an unbroken cycle -- as follows: 40 days . . . 40 days . . . 40 days . . . (and so on and on).
Of significance here is that each passing tropical year can effectively be metered out by simply tracking 40 days (as an unbroken cycle).
The cited measurement of the tropical year only requires that 39 days in each cycle of 40 days be exclusively counted. (Hint: This count can achieve a sum of 356 days per year).
The following diagram more clearly illustrates that -- as long as 40th days are never counted -- the revolution of each solar year can exactly be paced by an exclusive count of the other days:
__________________________________ A PERFECT 9-YEAR CALENDAR * __________________________________ Renewal = 1 day Year 1 = 356 days Year 2 = 356 days Year 3 = 356 days Year 4 = 356 days Year 5 = 356 days Year 6 = 356 days Year 7 = 356 days Year 8 = 356 days Year 9 = 356 days * Each 40th day (a perpetual rate) must be leaped or intercalated apart from days comprising the the cited calendar count. __________________________________ Calendar = 3205.00000 days 40 days uncounted = 82.17949 days 9 year average = 3287.17949 days 1 year average = 365.24217 days Solar-year rate = 365.24219 days Average difference = 2 seconds
The diagram shows that each passing solar year can very effectively be measured and metered out in correspondence with a numbered count of 356 days -- when 40th days throughout the time stream are never numbered among the other days.
An exclusive count of 3205 days in association with the passage of 9 solar circles requires the perpetual reckoning of 'unbroken cycles'. In essence, the track of a cycle of 40 days must never be preempted.
The cited calendar count of 9 years inherently aligns with each time segment of 9 tropical years (on the average). This equivalency is easy to recognize in that the rate of 1 day in 40 days is equal to 2.5 percent of time and the rate of 3205 days in 9 years is equal to 97.5 percent of time. Thus, in combination, these two rates of days can exactly account for all of time (100 percent).
In this modern era, the track of a 40-day cycle can be used to determine the epoch of each passing solar year to within the limits of 2 seconds (on average). However, because Earth's spin appears to be slowing down by a tiny amount with each passing century, it can be recognized that the track of a 40-day cycle could have been used to exactly (perfectly!) measure and meter the solar-year rate in some era of the past. The era when the solar year could have been perfectly measured and metered out using a track of 40 days was probably within very recent centuries.
The above cited count of 40 days is additionally significant in that each passing year can also be metered and measured by counting out a somewhat different primary cycle.
To be more specific about another method by which each passing year can be measured, a cycle of 9 years can effectively be time tracked within the context of counting a cycle of 360 days (as a primary cycle). In tandem with counting a primary cycle of 360 days, a calendar count of 360 days is also performed in parallel. The purpose of the secondary count of 360 days is to define/delimit a set of days that keeps pace with the traverse of each tropical year.
A double count of 360 days can achieve an accurate annual track as long the following rules are applied:
- From day-to-day, a primary cycle of 360 days is counted in repetition (as a primary, unbroken cycle).
- From year-to-year, a secondary calendar count of 360 days is performed (to keep pace with each annual revolution).
- Upon completion of every quarter of the cited primary count of 360 days, the secondary count of 360-days must be suspended for a day. In addition, the secondary count of 360 days must be suspended for a day in those years when the 4 quarters of the primary cycle come into alignment with the calendar year.
- Upon each full revolution of the primary cycle of 360 days, the secondary calendar count of 360 days must be suspended for a day.
The above stated rules are required to properly intercalate an annual calendar of 360 days. The set of rules could also be stated in terms of counting a 90 day cycle. For example, a day could be added to the calendar at a frequency of each 90 days, while yet an additional day could be added to the calendar at a frequency of each 360 days.
The rules required to intercalate a 360-day calendar could, in the most simple form, be expressed in the context of a rate of 5 days for every complete revolution of the primary time cycle (360 days).
Obviously, more than a single set of rules would be apropos for intercalating a calendar of 360 days. In any case, a base rate of intercalation equal to 5 days for each time segment of 360 days would be required.
From the given detail of the current model it should be clear that no other adjustment is required other than the addition of 5 days upon the completion of each primary cycle of 360 days. Also, the addition of 1 more day is required in those occasional years that contain all 5 quarter nodes of the primary count.
Thus, a precise 360-day calendar can be derived from out of the time track of an endless cycle of 360 days. The cited double counts function perfectly together in that a primary cycle (360 days) is used to define/delimit an exclusive set of days each year. The additionally defined days each year, normally 5 days, are simply not counted like the other calendar days (360 days in each tropical year).
The following diagram shows that the time traverse of each tropical year can effectively be measured and metered out by tracking primary and secondary cycles of 360 days -- where the secondary cycle represents a calendar count that is preempted by the inclusion of additional days:
_____________________________________ A PERFECT 9-YEAR CALENDAR * _____________________________________ Renewal = 1 day Year 1 = 360 days Year 2 = 360 days Year 3 = 360 days Year 4 = 360 days Year 5 = 360 days Year 6 = 360 days Year 7 = 360 days Year 8 = 360 days Year 9 = 360 days * - 5 days are added every 360 days and 1 more day is added to years containing a 5th quarter node. ____________________________________ Average calendar = 365.24217 days Solar-year = 365.24219 days Average difference = 2 seconds
Of course, the 360-day model as diagrammed, clocks to the very same high degree of accuracy as the above cited 40-day calendar (a calendar predicated upon a cycle of 40 days). Again, it seems of some certain significance that the accounting of only two short cycles can achieve what is probably the very most accurate calendar that can be derived from out of the spin and orbital phenomenon.
Of significance here is that several of the old-world calendars appear to have been predicated upon the revolution of 360 days. In some of the systems that used a 360-day count, intercalation of 5 days was deferred beyond the limits of a single year. For example the Kultepe Calendar appears to have been predicated upon a count of 10 days. This count (ten) was repeated across a time grid of 30-day cycles. "For three years, the ten counts ran congruently with the months and the years. Then, after the insertion of a 15-day shapattum period, they [the '10 counts'] overlapped from one month into the next, returning to congruency with the months after the next shapattum... " (Britannica, 1972).
For more information about a primary count of 360 days, refer to the following online publications:
Significance of a 7 day count
The limits of each passing tropical year can additionally be determined (on the average) by simply keeping track of a cycle of 7 days.
Of significance here is that Jewish literature produced in the era of the Second-Temple does mirror that a segment of early astronomers did time track the tropical year in correspondence with a count of 364 days or stations -- which is 52 weeks:
"... the exactness of the year is accomplished through its separate three hundred and sixty-four stations." ('Enoch', Chapter 75: 2-3, translated by R.H. Charles).
Note that most of the early sources indicate that the length of the year was counted out using a fixed count of 364 days:
- "And the year is complete - three hundred and si[xty-four] days" (refer to Scroll 4QMMT, lines 20-21).
- "On that very day, Noah went from the ark at the end of a complete year of three hundred and sixty four days, on the first (day) of the week... " (refer to Scroll 4Q252 2:2-3).
- "... Four [seasons]... divide the four portions of the year... they belong to the reckoning of the year... one [seasonal division] in the first portal and one in the third, and one in the fourth and one in the sixth, and the year is completed in three hundred and sixty-four days." ('Enoch', Chapter 82:4-6, translated by R.H. Charles).
It is significant that the cited annual count of 364 days was sometimes represented as 52 weeks of days. (Note that 52 weeks per year at 7 days per week is equal to an annual count of 364 days).
"And all the days of the commandment will be two and fifty weeks of days, and (these will make) the entire year complete... observe the years according to this reckoning - three hundred and sixty-four days... [You must] make the year three hundred and sixty-four days only... " ('Jubilees', Chapter 6:30-38; translated by R.H. Charles).
Because a 364-day calendar is shorter in length than the solar year of 365.24 days, it becomes a given conclusion that the cited calendar of weeks would have required periodic intercalation. This then means that certain of the calendar years would have required the insertion of a leap week.
The intercalation of a week would have been necessary in certain of the years so as to keep a calendar of 364 days (the length of 52-week units) properly aligned with the solar year (which is 1.24 days longer than 364 days).
It is here significant that a calendar comprised of 7-day units can be recognized to additionally define a uniform grid of 7-year units. A greater calendar grid comprised of 7-year cycles is inherent from the requirement to add a leap week at the interval of each 7th year (as is futher shown below).
The continuous track of both time cycles (7 days and 7 years) can be recited from numerous passages of Second-Temple literature. For example, 'Antiquities of the Jews' has: "[The Judeans rest] every seventh year, just as on the seventh day." (Book 13:8:1); 'Wars of the Jews' also has: "the Jews rest every seventh year as they do on every seventh day"; and 'The Book of Jubilees' shows that "[Enoch] recounted the weeks of the jubilees... and recounted the Sabbaths of the years... " (Chapter 4:18).
The following diagram shows that a sequence of 7-year cycles is easy to interpret from out of an ongoing count of 7-day cycles (52 weeks per year). Take note that a distinct 7-year cycle is inherently defined from out of the requirement to intercalate a week at always the distance of 7 solar years. The respective leap week--required at each 7-year interval as diagrammed--is fully necessary so as to interface the fixed rate of 52 weeks (or the rate of 364 days) with the longer rate of the annual circle (which is 365.24 days).
___________________________________ A Perpetual Interface * (52 Weeks Per Year) ___________________________________ 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week 7 years = 7 times 52 weeks + 1 Week At Each 70 Years... + 1 Week ___________________________________ * - Requires intercalation of an additional week in a loop at 7 sets of 7 years.
A given conclusion from the indicated calendar count of 364 days (or 52 weeks) per year then is that additional intercalation would have been necessary to keep the stated calendar of weeks in pace with the length of the tropical year (365.24 days). Remarkable here is that the required rate of calendar intercalation does inherently overlay a time grid of 7 years.
Thus, an effective annual calendar (which averages 364.24 days over time) is possible through a simple accounting of 52 weeks per year. According to this respective interpretation of an annual calendar, the uniform rate of a cycle of 7 days is used to ultimately define sets of 7 years in long-cycle segments.
A count of the 'weeks-of-days' across 'weeks-of-years' represents an extremely effective method of determining the length of the annual circle (refer to the previous diagram). Note from the rates expressed in the diagram that 52 weeks per year times 7 days per week is equal to 364 days in each annual cycle. The addition of 1 more week at time intervals of 7 years, 49 years, and 70 years equates to the following day rates: 7 days in 7 years is equal to 1.00 more day per year on the average; 7 days in 49 years is equal to 0.14286 more day per year on the average; and 7 days in 70 years is equal to 0.10 more day per year on the average. Thus, the average annual rate of the cited calendar of weeks inherently is 364 days + 1.00 + 0.1429 + 0.10 days = 365.2429 days. This annual calendar rate compares very closely with the actual solar year of 365.2422 days (by less than 1 minute per solar year). For additional information, refer to the online publication:
A day count for tracking the zodiac
Several interpretataions for 'day counting' 12 annual divisions are possible. One of these interpretations sees a tally of 48 weeks being preempted 6 times per year by the inclusion of an additional day (as a single, non-week day).
The subsequently presented diagram illustrates the feasibility of performing an annual day count. Note here that the occurrence of the cited 6 annual days per year (6 portal days) are determined at the distance of 2 zodiacal divisions for each day.
------------------------------------ A CALENDAR OF PORTALS AND WEEKS --------- ---------- ------------- Portal Zodiac Solar Month Days Month Days --------- ---------- ------------- 1 1 7 + 7 + 7 + 7 2 7 + 7 + 7 + 7 1 3 7 + 7 + 7 + 7 4 7 + 7 + 7 + 7 1 5 7 + 7 + 7 + 7 6 7 + 7 + 7 + 7 1 7 7 + 7 + 7 + 7 8 7 + 7 + 7 + 7 1 9 7 + 7 + 7 + 7 10 7 + 7 + 7 + 7 1 11 7 + 7 + 7 + 7 12 7 + 7 + 7 + 7 --------- ---------- ------------- 6 days 336 days ------------------------------------ The cited calendar of 48 weeks inherently paces the return of the year when additional weeks are intercalated--as follows: 1. At 7th months (+ 1 week). 2. At 7th pentecontads (+ 1 week). 3. At 7th seasons (+1 week).
Note that a grid of 48 weeks (a calendar of weeks as diagrammed) can pace the return rate of each passing tropical year as long as the count of an additional week is routinely included (or intercalated).
Significant about the cited rate of 48 weeks (with added weeks) is that the resulting calendar rate averages out to be equal to 365.24232 days on an annual basis. To be specific, a count of 342 days can be correlated to each annual circle when the other days are accounting for in the context of the following time cycles:
- 1 additional week at every 7th month of 30 days. [The count rate of this cycle is equal to 12.17473 days per year.]
- 1 additional week every 7th pentecontad, where a pentecontad is a time span equal to 7 lunar quarters. [The count rate of this cycle is equal to 7.06758 days per year.]
- 1 additional week every 7th season. [The count rate of this cycle is equal to 4.00000 days per year.]
The cited rate of 342 days with additional week cycles is then equal to a composite rate of 365.24232 days per year (rounded from expanded precision). Thus, the shown calendar model renews (on the average) right in association with each passing tropical year (of 365.24219 days).
Of significance here is that the rate of the modern tropical year clocks at a tiny difference away from the average return of the cited weeks calendar. It is here significant that a difference of less than 1 second per month can be recited. However, the spin rate of the Earth appears to be slowing down by a fractional amount in correspondence with each passing century. The slowing spin factor thus indicates that the return of the tropical year would recently have been EXACTLY synchronized with a fixed count of weeks (as diagrammed and documented).
The Earth inherently spins 365 times per year, and the spin rate throughout recent millennia has slowed down at a rate of between 0.001 and 0.003 seconds per century. A given conclusion from these respective rates then follows: 1. A loss in the annual definition of at least 0.365 spin-seconds has been experienced (a per-century rate); and 2. The rate of 1 second of modern difference per month (12 seconds per year) when divided by a gain of 0.365 spin-seconds per century, points to a time of no (zero) difference with a calendar of weeks at only 33 centuries ago.
The feasibility of reckoning 6 distributed annual days amid a calendar of weeks is more fully detailed in an online publication entitled:
As a variation of the model shown above, the following diagram shows that an effective calendar for pacing 48 annual divisions can likewise be achieved by likewise tracking a completely unbroken cycle of 7 days:
------------------------------------ A CALENDAR OF WEEKS IS POSSIBLE (48 Weeks per Tropical Year) ---------- ------------- Zodiac Solar Month Month Days ---------- ------------- 1 7 + 7 + 7 + 7 2 7 + 7 + 7 + 7 3 7 + 7 + 7 + 7 4 7 + 7 + 7 + 7 5 7 + 7 + 7 + 7 6 7 + 7 + 7 + 7 7 7 + 7 + 7 + 7 8 7 + 7 + 7 + 7 9 7 + 7 + 7 + 7 10 7 + 7 + 7 + 7 11 7 + 7 + 7 + 7 12 7 + 7 + 7 + 7 ---------- ------------- 336 days ------------------------------------ The depicted calendar of weeks does inherently pace the return of each passing year as long as additional weeks are intercalated--as follows: 1. At 7th months (+ 1 week). 2. At 7th pentecontads (+ 1 week). 3. At 7th portals (+1 week). 3. At 7th seasons (+1 week).
This respective calendar of weeks achieves the same accuracy (in average time) as the calendar previously presented. Again, the week cycle of 7 days is here integral in the defining 48 divisions throughout each rotation of the tropical year.
The following diagram is illustrative of yet another weeks calendar. This respective interpretation sees the annual return as being subdivided in 7 divisions. Yet again, each tropical year can perfectly be metered and measured out (on the average) by simply accounting for week cycles (where each passing week corresponds to an endless cycle of 7 days):
------------------------------------- A CALENDAR OF WEEKS IS POSSIBLE (49 Weeks per Tropical Year) --------- -------------------- Annual Annual Divisions Weeks --------- -------------------- 1 1 2 3 4 5 6 7 2 8 9 10 11 12 13 14 3 15 16 17 18 19 20 21 4 22 23 24 25 26 27 28 5 29 30 31 32 33 34 35 6 36 37 38 39 40 41 42 7 43 44 45 46 47 48 49 --------- -------------------- 343 days ------------------------------------- The depicted calendar of weeks does inherently pace the return of each passing year as long as additional weeks are intercalated--as follows: 1. At 7 months (+ 1 week). 2. At 7th lunar pentecontad (+ 1 week). 3. At 28th zodiac division (+ 1 week).
The previously shown calendar of weeks achieves the same accuracy (in average time) as calendars previously presented.
Note that 343 days and 12.17473 days and 7.06758 days and 3.00000 days are equal to 365.24232 days per year (rounded from expanded precision).
The indicated required addition of 7 days at every 7th month, every 7th pentecontad, and every 28th zodiac division then does quite perfectly correspond with a rate that is necessary in defining/delimiting an annual set of 49 weeks.
The previous sections have attempted to show that the Earth-Moon can be interpreted to represent an interrelated system. It is here significant that both the synodic month and the solar year alike appear to share a same peculiar common denominator--which is the length of the lunar-quarter or the lunar week. Note that the lunar quarter or lunar week reoccurs in a time interval of 7.38265 days--over average time. Fixed counts of 7 lunar weeks inherently define a very specific boundary that is fully necessary for aligning day counts with the passage of the seasons and ultimately with the revolution of the year. The resulting alignment of the solar day with the tropical year can thus ultimately be interpreted as some very good evidence of interrelated time design. (Remarkable here is that passages in the Bible and related texts have explicit detail of a 7 week count followed by the celebration of a special day -- the Asartha). For additional information concerning interrelatedness of the Earth-Moon, refer to the following online articles:
_________________________
Changes Throughout Time
Current measurements of the Earth-Moon system reveal that the rate of the rotation of the Earth is gradually slowing down. (Obviously, this gradual shift ultimately will alter existing interrelationships as time progresses).
The current and subsequent chapters will supply information concerning how design is altered by the passage of time, and specifically will detail the phenomenon of a gradual change in the length of the day.
Modern estimates
Reliable almanac statistics for the Sun and the Moon can be computed from data collected at a fixed location on the globe (such as at an astronomical observatory).
Over average time the Sun rises according to a regular interval of 24 hours (actually a tiny fraction different from 24 hours as further explained below). Also over average time, the Moon passes through a complete synodic cycle in 29.53059 solar days, and the annual cycle routinely completes each 365.2422 days.
It is pertinent to note, based upon modern measurements, that these rates are slowly changing with time. It seems over average time that the rotational spin of the Earth is slowing down by a fractional amount each century. This equates to a fractional increase in the length of the day with each passing century (and a simultaneous fractional decrease in the day counts of the lunar and annual cycles--as further explained below).
According to the U.S. Naval Observatory (Time Services:
"The Earth is constantly undergoing a deceleration caused by the braking action of the tides. Through the use of ancient observations of eclipses, it is possible to determine the deceleration of the Earth to be roughly 1-3 milliseconds per day per century. This is an effect which causes the Earth's rotational time to slow with respect to the atomic clock time. Since it has been nearly 1 century since the defining epoch (i.e. the ninety year difference between 1990 and 1900), the difference is roughly 2 miliseconds per day. Other factors also affect the Earth, some in unpredictable ways, so that it is necessary to monitor the Earth's rotation continuously... ".
This indicated very small change in the rotational spin of the Earth is meticulously monitored by the International Earth Rotation Service (IERS) in Paris. According to the IERS:
"Universal time and length of day [LOD] are subject to variations due to the zonal tides (smaller than 2.5 ms in absolute value), to oceanic tides (smaller than 0.03 ms in absolute value), to atmospheric circulation, to internal effects and to transfer of angular momentum to the Moon orbital motion."
Determining time on a spinning Earth
The changing spin of the Earth can be verified from atomic clock measurements.
Atomic clocks were first put into wide use only a few decades ago and within this short span of time it has been necessary to insert a number of leap seconds into civil time based upon the atomic clock (TAI).
According to the U.S. Naval Observatory (Time Services):
"In order to keep the cumulative difference... less than 0.9 seconds, a leap second is added to the atomic time to decrease the difference between the two. This leap second can be either positive or negative depending on the Earth's rotation. Since the first leap second in 1972, all leap seconds have been positive. This reflects the general slowing trend of the Earth due to tidal braking.
Confusion sometimes arises over the misconception that the regular insertion of leap seconds every few years indicates that the Earth should stop rotating within a few millennia. The confusion arises because some mistake leap seconds for a measure of the rate at which the Earth is slowing. The one-second increments are, however, indications of the accumulated difference in time between the two systems. As an example, the situation is similar to what would happen if a person owned a watch that lost two seconds per day. If it were set to a perfect clock today, the watch would be found to be slow by two seconds tomorrow. At the end of a month, the watch will be roughly a minute in error (thirty days of two second error accumulated each day). The person would then find it convenient to reset the watch by one minute to have the correct time again.
This scenario is analogous to that encountered with the leap second. The difference is that instead of setting the clock that is running slow, we choose to set the clock that is keeping a uniform, precise time. The reason for this is that we can change the time on an atomic clock while it is not possible to alter the Earth's rotational speed to match the atomic clocks! Currently the Earth runs slow at roughly 2 milliseconds per day. After 500 days, the difference between the Earth rotation time and the atomic time would be one second. Instead of allowing this to happen, a leap second is inserted to bring the two times closer together."
The following table is based upon atomic clock measurements and shows the number of leap seconds required into civil time. These leap seconds are based upon a difference in the rate of the rotation of the Earth and a constant time based upon atomic clocks.
__________________________________ Date Of Cumulative Seconds Leap Second * (UTI minus TAI) ___________ __________________ 1961 JAN 1 1.4228180 1961 AUG 1 1.3728180 1962 JAN 1 1.8458580 1963 NOV 1 1.9458580 1964 JAN 1 3.2401300 1964 APR 1 3.3401300 1964 SEP 1 3.4401300 1965 JAN 1 3.5401300 1965 MAR 1 3.6401300 1965 JUL 1 3.7401300 1965 SEP 1 3.8401300 1966 JAN 1 4.3131700 1968 FEB 1 4.2131700 1972 JAN 1 10.0 1972 JUL 1 11.0 1973 JAN 1 12.0 1974 JAN 1 13.0 1975 JAN 1 14.0 1976 JAN 1 15.0 1977 JAN 1 16.0 1978 JAN 1 17.0 1979 JAN 1 18.0 1980 JAN 1 19.0 1981 JUL 1 20.0 1982 JUL 1 21.0 1983 JUL 1 22.0 1985 JUL 1 23.0 1988 JAN 1 24.0 1990 JAN 1 25.0 1991 JAN 1 26.0 1992 JUL 1 27.0 1993 JUL 1 28.0 1994 JUL 1 29.0 1996 JAN 1 30.0 1997 JUL 1 31.0 1999 JAN 1 32.0 __________________________________ * -- IERS data
Essentially, based upon the rate of the rotation of the Earth (and atomic clock measurements), thirty-two leap seconds have been determined in comparison with the precise definition of the length of one second. This precise definition of the second is oriented to the epoch (which is the beginning of the twentieth century).
The following paragraph--borrowed from the Naval Observatory--explains the specific definition of one second (and the peculiar epoch at the beginning of the twentieth century):
"Civil time is occasionally adjusted by one second increments to insure that the difference between a uniform time scale defined by atomic clocks does not differ from the Earth's rotational time by more than 0.9 seconds. Coordinated Universal Time (UTC), an atomic time, is the basis for our civil time.
In 1956, following several years of work, two astronomers at the U.S. Naval Observatory (USNO) and two astronomers at the National Physical Laboratory (Teddington, England) determined the relationship between the frequency of the cesium atom (the standard of time) and the rotation of the Earth at a particular epoch. As a result, they defined the second of atomic time as the length of time required for 9,192,631,770 cycles of radiation corresponding to the transition between two hyperfine levels of the ground state of the cesium 133 atom at zero magnetic field.
The second thus defined was equivalent to the second defined by the fraction 1/31,556,925.9747 of the year 1900. The atomic second was set equal, then, to an average second of Earth rotation time near the turn of the 20th century."
The rotation of the Earth since the turn of the twentieth century is thus indicated to be slowing down. Throughout this century, the length of the day has increased by an average amount of about 0.002 seconds. This seems to be a very small amount of time, but--because this increase applies to a daily spin rate (where currently in one revolution a time difference of about 2 milliseconds is produced... and in two revolutions a time difference of 4 milliseconds is produced... and in three revolutions a time difference of 6 milliseconds is produced... and so on)--this time difference cumulates day-after-day, and as time progresses, a respective position on the globe requires a greater and greater amount of time to 'catch-up' with the same position in the past.
If during the run of the next ten centuries (or one millennium), the length of the day hypothetically increased at the rate of 0.002 seconds per century then this increase becomes equal to 0.020 seconds of increase by the end of the one-thousand years (0.002 seconds per century times 10 centuries is equal to 0.020 seconds of increase). Because there are roughly 365,000 spins of the Earth in one thousand years then the total number of catch-up seconds required become equal to 4,015 seconds as shown in the following diagram:
__________________________________ Century Increase Number Century Date In Length of Days Increase of Day in the Seconds (Seconds) Century ______ _________ _______ _______ 2000 0.002 36,500 73 2100 0.004 36,500 146 2200 0.006 36,500 219 2300 0.008 36,500 292 2400 0.010 36,500 365 2500 0.012 36,500 438 2600 0.014 36,500 511 2700 0.016 36,500 584 2800 0.018 36,500 657 2900 0.020 36,500 730 ___________________________________ Time Span = 10 Centuries Day increase = 0.020 Seconds Spins (Days) = 365,242 Days Time increase = 4,015 Seconds
As this hypothetical example illustrates, a slow down in the daily rotation of the Earth at the rate of 0.002 seconds per century seems small and insignificant, but this small change has a very significant cumulative effect over time. In the cited example, 4,015 seconds (or 1 hour, 6 minutes, and 55 seconds) would need to be leaped from civil time (in order to keep the rotation of the Earth positioned into a 24 hour clock window). (For additional information, refer to the Appendices).
Determining the spin of Earth before atomic clocks
It should be clear from information presented in the section above that modern clocks are based exactly upon 24 hours per day (or 86,400 seconds per day) and that the rotation of the Earth currently expires a bit in excess of 24 hours per day. Thus, atomic clocks--oriented to the precise definition of one second--define each day to be precisely 86,400 seconds--and yet the Earth's rotation doesn't exactly keep pace with the clock.
This deviation throughout the current twentieth century raises a question concerning how much the Earth's spin has changed throughout previous centuries and millennia?
Obviously, atomic clock data isn't available from centuries gone by. Rather than clock data, eclipse data is available. (Note that the record of an eclipse from a specific location on the globe reflects the time and location of a prior Earth and Moon alignment).
It is here significant that modern astronomers have studied hundreds of ancient eclipses. The times and the locations of these prior Earth-Moon alignments adequately reflect that the spin of the Earth has slowed throughout prior millennia.
Stephenson and collaborators have produced numerous publications concerning ancient eclipses and the slowing of Earth's spin. For more information concerning modern research into historic eclipses, refer to Appendix C.
Determining the spin of Earth before historic times
Some scientists believe that the rate of the Earth's rotation can be determined all the way back into previous geologic ages based upon coral records. Essentially, because coral keeps a record of how much it grows (like tree rings) then the coral growth record can be used to determine when it grew and by how much.
For example, coral from Pennsylvanian rock beds have about 387 daily layers per year, while coral from the Devonian rock beds have about 400 daily layers per year.
This indicates that from approximately 300 to 400 million years ago the annual cycle ranged from 387 to 400 days in length (an annual cycle strangely different from the present annual cycle of 365 days).
The recent Moon
Based upon modern measurements, and also based upon the occurrences of eclipses, contemporary astronomers have been able to catalog historic phases of the Moon.
A catalog of Moon phases presented at the NASA Eclipse Web Site shows that (throughout recent Millennia) the length of the synodic month can be equated to about 29.53060 days (on the average). The historic period of the Moon is consequently about the same as the modern period.
For pertinent information concerning the historic definition of the synodic month, refer to the subsequently presented Appendix F.The current Earth-Moon configuration doesn't have to mean the non-existence of a more distant history. In essence, previous eras may have came and gone before (well before the arrival of humans). For additional information on prior changes in the Earth-Moon configuration, refer to the online document entitled:
________________________
Functional Composition
When viewed from the Earth, the Sun and the planets appear to rise and set (always from east to west).
The Moon appears to shine, but in actuality its surface is reflecting light from the Sun. The "shining" portion of the lunar surface--as viewed from the Earth--changes night-after-night (as the illuminated portion of the Moon's surface waxes and wanes according to a periodic cycle known as the lunar-month cycle).
As the Moon celebrates light from the Sun--in the waxing half of the lunar-month cycle--the lunar surface increases in luminance for two weeks before becoming wholly illuminated (at the full phase). In the waning half of the lunar-month cycle, the lunar surface subsequently decreases in luminance for two weeks before becoming wholly dark (at the new phase).
The lunar-month cycle--referred to as the synodic month--completes in 29.5 days. The actual obit of the Moon around the Earth is called the sidereal month and completes in 27.3 days. The synodic month is longer than the sidereal month because the Earth is moving around the Sun and the relative positions of Earth and the Sun throughout 27 days are altered, and consequently the Moon must travel farther in order to come again into alignment (between the Earth and the Sun). Thus, from the perspective of an observer stationed on the Earth, the synodic month, or lunar month (of 29.5 days) is the apparent or perceived lunar cycle and is therefore significant to the observer.
It is significant that the annual cycle passes through four distinct seasons (in each solar cycle), and likewise, the lunar-month cycle passes through four distinct quarter phases. The four phases of the lunar-month cycle are: (1) New phase; (2) First-quarter phase; (3) Full phase; and: (4) Last-quarter phase.

The lunar-month cycle first begins when the Moon moves closest to the Sun. At this time the Moon appears to be dark (with no portion of its surface reflecting any light from the Sun). The Moon--as viewed from the Earth--is at its new phase on this special day. Night-after-night more of the crescent shaped Moon is illuminated as it appears after dusk higher-and-higher in the western sky. After one week of waxing, the Moon--at dusk--appears directly overhead between the western and eastern horizons. At this time, the Moon arrives at its distinct first-quarter phase. At the first-quarter phase, the Moon is no longer crescent shaped, and it appears one-half illuminated (exactly like the letter 'D'). Following the first-quarter phase, the Moon, night-after-night, continues to wax in luminance and at dusk it appears closer and closer to the eastern horizon. Seven days following the first-quarter phase, the Moon appears on the eastern horizon at dusk, and its surface now appears wholly illuminated (the full phase). When the Moon is fully illuminated (the full phase), the Moon appears between the eastern and western horizons for an entire evening of viewing. After the unique full phase, the Moon begins to wane and--night-after-night--less of the Moon is illuminated. Also, the Moon rises from the eastern horizon about fifty minutes later evening-after-evening. After a week of waning, the Moon arrives at its distinct third-quarter phase. At this time, the Moon again appears one-half illuminated but the illuminated half appears in reverse from the first-quarter phase (like a backwards letter 'D'). At the third-quarter phase, the Moon doesn't rise from the eastern horizon until the middle of the nighttime. Following the third-quarter phase, the Moon again becomes crescent shaped, and less-and-less of the crescent shaped Moon becomes illuminated as it continues to wane night-after-night. Seven days following the third-quarter phase (or the last-quarter phase), the Moon arrives once again at its special new phase.
The four distinct quarter phases of the Moon can be predicted to appear throughout the four seasons of the year (as described below):
- New phase (rides high in sky in summer and low in winter, and it reaches an intermediate height in spring and fall).
- First-quarter phase (rides low in the fall and high in the spring, and it takes a middle course during summer and winter).
- Full phase (rides low in summer, the same as the Sun at noon in midwinter; and rides high in winter, comparable with that of the Sun at noon in the summer; and it takes an intermediate height in spring and fall).
- Last-quarter phase or third-quarter phase (rides high in the fall and low in the spring, and it follows an intermediate height in summer and winter).
The significance of Earth's environment
As is cited above, processes which are apparent to an observer stationed upon the Earth--such as day and night, lunar phases, lunar months, seasons, and annual cycles--may not be very apparent to an observer stationed in space (and from the perspective of an observer stationed in space, what is real in the solar system--such as the size of the Sun and Moon, sidereal days, sidereal months, and sidereal years--would be perceived very differently than to an observer stationed on the Earth).
Subsequent paragraphs and sections will then attempt to show more of how significant Earth's environment appears to be for interpreting the apparent Moon and Sun.
As an example, the Earth's rotational axis happens to be tilted at 23.5 degrees relative to the Sun's orbit. As the Earth orbits around the Sun, an Earth's resident experiences seasonal differences due to the cited 23.5 degree tilt.
Another example of the significance of Earth's environment concerns the Moon's apparent diameter--where, from the Earth, the Moon appears to have the same size diameter as the Sun's diameter. Essentially, to an observer stationed on the Earth, both orbs (the Sun and the Moon) appear to have the same angular size (of 0.5 degrees).
Equal angular sizes--perceivable only to an observer stationed on the Earth--seems to reflect that both the Sun and the Moon are specifically positioned (relative to the Earth).
The phenomenon of equal Moon and Sun sizes--whereby both orbs appear to have the same angular size--is integral in the periodic formation of a solar eclipse. It is here significant that the orbit of the Moon happens to be tilted at only 5 degrees with the Sun. The peculiar angle of the Moon orbit (in close alignment with the Sun) is ultimately necessary for the formation of a solar eclipse on the Earth. (If the orbit of the Moon did not closely align with the Sun then the Moon would never move into direct position between the Sun and the Earth; and consequently, a solar eclipse would never occur).
The Earth/Moon/Sun configuration is such that solar eclipses can only occur at the appearance of the new phase of the Moon, and lunar eclipses can only occur at the appearance of the full phase of Moon. The combined number of eclipses (including partial eclipses) of the Sun and the Moon cannot be less than two, or be more than seven, in a calendar year. Solar eclipses repeat their cycle every 223 lunar months.
It then seems more than coincidental that for a total eclipse of the Sun to be observable from the Earth, the Moon's apparent diameter must equal or exceed the Sun's apparent diameter (and this is so--as explained above). In addition, the angle of the Moon's orbit must uniquely align with Earth's orbital plane around the Sun (and this also is so!).
So the formation of a solar eclipse requires the right combination of diameter sizes, relative positions, and tilt of orbits. This correct combination happens to exist--and even in concert with the 23.5 degree tilt of the Earth's axis.
Of possible significance to a study of functional time design is that the cited saros cycle of 223 synodic months can effectively be metered out using a lunar-weeks count (or a lunar-quarter count) of 99 times 9 plus 1.
The Moon's rotational rate
A mystery concerns the Moon and its rotational period.
The sidereal orbital period of the Moon around the Earth completes in 27.321666 days while the rotational period of the Moon also completes in 27.321666 days! In essence, the Moon rotates once upon its axis every time it orbits around the Earth.
This means that as the Moon progresses throughout its orbit about the Earth the same side of the Moon shows toward the Earth at all times. (If the Moon did not rotate upon its axis in synchronization with its orbit then its opposite side would eventually revolve into view from the Earth).
Due to the Moon's synchronized orbit and rotation, only about 59 percent of its surface is ever visible from the Earth. (Note that a bit more then one-half of the normal face of the Moon is visible throughout time because of the Moon's slightly elliptical orbit). This tipping face of the Moon is known as "libration in longitude" and as "libration in latitude"--where over a long period of time "libration" permits the viewer to see a little bit farther around the edges of the normal face of the Moon.
It isn't known just why the Moon rotates in correspondence with a synchronized orbital rate but it is possible that the cited synchronization is the result of Earth's gravitational pull--where the diameter of the Moon is estimated to be bulged toward the Earth (by the amount of about one-third mile).
Thus, a mysterious tie or connection seems to exist between the Earth and the shining Moon--which perpetually faces the Earth in its continuous orbit.
Moon geography
The geography of the Moon consists of level plains, valleys, and mountain peaks (similar to those on the Earth) but it possesses many geological features which are different.
The features viewed as "the man in the Moon" are a combination of craters, mountain peaks, deep narrow valleys, and level plains, or maria. The largest of the maria is... about 700 miles in diameter. There are some 20 other prominent maria on the side of the Moon that faces the Earth... The maria are surrounded by huge mountains... with peaks up to 30,000 feet. Tens of thousands of craters are scattered over the Moon's surface, often overlapping one another... [Some] craters have rays. These are light-hued lines radiating from the craters like the spokes of a wheel. Some rays are more than 1,000 miles long. None is more than about 12 miles wide... The large craters range from less than a mile to nearly 150 miles in diameter. Like the maria, they are surrounded by high peaks. There are more than a thousand deep valleys, called rills, or clefts, on the Moon. They are 10 to 300 miles long and 2 miles or less wide... depths are unknown... During a solar eclipse, Sunlight shining down valleys on the edge of the Moon may form a circle of bright points known as Baily's beads... " (Compton's Encyclopedia, 1995).
The geography of the Moon is somewhat unique in the regard that the near side of the Moon (or the side of the Moon that continuously faces the Earth) has geological features which differ from those of the far side of the Moon (or the side of the Moon that perpetually faces away from the Earth).
A number of lunar maps (both shaded relief maps and geologic maps), are available. (Maps can be ordered from USGS Information Services).
The far side of the Moon lacks the large flat places (or the maria) that appear on the near side of the Moon.
The largest flat spot on the Moon is known as Mare Imbrium (Sea of Rains). This flat place happens to be about 1200 km (or 750 miles) wide. This huge flat place, and a number of other prominent features, can be viewed and recognized from the Earth without the aid of magnified viewing.
Another facet about the unusual geography of the Moon is that the far side of the Moon is lighter colored.
Thus, the features that appear on the near side of the Moon--or the features that can be viewed from the Earth--are somewhat unusual in comparison with those that cannot be seen on the far side of the Moon.
The Earth-Moon arrangement can be recognized to be unusual. In its ongoing celebration of light from the Sun, not only does the same side of the Moon perpetually face the Earth but the features of the Moon that can be viewed from the Earth appear to differ from the opposite side.
The size and orbit of the Moon
The Moon has a large comparative size relative to the size of the Earth--where the Earth is almost 8,000 miles in diameter, and the Moon's diameter is some 2,160 miles. The size of the Moon is therefore about one-fourth the diameter of the Earth, and this comparative size is larger than for any of the other planets in our solar system.
The Moon is not as dense as the Earth (about 3/5 as dense), and it only has about 1/81 the mass of the Earth.
The Moon's diameter is only very slightly enlarged (by only one-third mile), and this implies that the Moon is solid below the surface (otherwise gravity would have produced a far greater effect upon its diameter).
The large comparative size of the Moon and its nearness to Earth affects tides on the Earth (which are largely caused by the pull of the Moon--rather than the pull of the Sun). The ratio of the tide-raising pull of the Moon to that of the Sun is 11 to 5.
The Sun tends to pull the Moon away from the Earth (when the Moon is between the Earth and the Sun) and the Sun tends to pull the Moon toward the Earth (when the Moon is on the far side of the Earth). The effects of this pull are called perturbations.
The orbit of the Moon is approximately circular. It is--however--slightly elliptical (221,600 miles at perigee, and 252,950 miles at apogee). At perigee the Moon is nearest the Earth, and when it reaches apogee the Moon is the most distant--where these exact distances vary from month to month.
The Moon's orbit does not align with the Earth's equator, but rather aligns more closely with the Earth's path around the Sun (the ecliptic)--where it intersects the plane of the ecliptic at an angle of only 5 degrees.
The two points at which the Moon each month crosses the plane of the ecliptic are called the lunar nodes.
The orbit of the Moon is a bit complicated in that it does not remain in a completely flat plane. Instead its orbital plane oscillates slowly. This movement is sometimes called the regression of the nodes, since the nodes move constantly westward around the ecliptic. A full cycle of oscillation requires about 230 months (or 18.61 years) to entirely complete.
Of possible significance to a study of functional time design is that the Moon inherently rises at a rate that is about 50 minutes slower than the rate of the solar day (a rate equal to 1.03505 days on the average). A time span of 230 synodic months can thus effectively be metered out in correspondence with a count of 9 times 9 times 9 times 9 rises plus 1 rise (or 6562 rises).
Due to the oscillation, the Moon in some years ranges farther north or south in the sky, and this oscillation ultimately accounts for the occurrences of eclipses.
The nearly circular orbit of the Moon is somewhat of a science mystery. The close proximity of this orbit to the Earth cannot be explained purely through a capture process... as if the Moon were somehow captured into Earth's orbit. Essentially, computer simulations indicate that the Earth isn't large enough to capture and hold a large Moon. (Even if the Moon were somehow captured and held, it is unlikely that a close circular orbit of the current angular momentum would result. It seems that during the process of a capture the Moon would simply crash into the Earth).
The origin of the Earth-Moon system
A number of theories have been set forth to explain the composition and configuration of the Earth-Moon system.
One of the popular early analyses was an attempt to document the Moon as a world that formed in Earth's orbit (simultaneously as the Earth formed). However, based largely upon information gained during the latter part of the twentieth century, it now seems that the lunar and the terrestrial histories have been quite different. (The Moon has been discovered to be less dense than the Earth... and it seems to be lacking an inner core of iron--as does the Earth).
Another hypothesis has been that the Moon was captured into orbit around the Earth. This theory appears to still be under investigation by some researchers; however, this respective theory no longer represents the mainstream view. It is believed that the Moon could not have been captured for the following primary reasons:
- The Moon's orbit is in close proximity to the Earth.
- Computer simulations indicate that capture or condensation could not produce the nearly circular orbits of the Earth and the Moon.
- Capture or condensation ideas do not explain why the Earth's axis is tipped 23.5 degrees.
- It is now known that lunar rocks have a strong similarity to materials found near the Earth's surface, and it seems unlikely that this material originated from somewhere else in space.
Another theory has investigated the possibility that in early stages the Earth flung off the Moon. However, studies by astrophysicists indicate that the escape of the Moon from the Earth is improbable (as it is most difficult to find mechanical processes by which an object such as the Moon could be removed from the Earth).
Throughout the last two and one-half decades, a new theory--the giant impact hypothesis--has grown in popularity. This theory is that the Moon was created when the primal Earth was hit by a huge fast moving body. (The Earth is believed to have been hit a glancing strike from a body larger than the size of Mars).
According to the giant impact hypothesis, an off-center strike was necessary to cast-off (or gouge-out) enough of the Earth's outer material to form the Moon. This glancing collision ejected Earth's material at speeds exceeding Earth's escape velocity (or 25,000 mph). Following this raking-off of the Earth's material, the large impacting body fragmented, and some of the cast-out material eventually formed a Moon in Earth's orbit.
The giant impact hypothesis is perhaps the best of all the theories to explain the near orbit of the Moon.
Based upon computer simulations, it seems that an off-center strike could have cast off enough of the Earth's outer material to form the Moon in close proximity to the Earth (and this explains the near orbit). This blow could also have produced about 18 degrees of the 23.5 degree tilt of the Earth (but subsequent impacts are required to ultimately achieve the additional 5 degrees of tilt).
A giant impact, if actually true, means that the Moon is composed from outer material from the Earth, and this is in part supported by modern knowledge of the composition of the Moon--which indicates the inner-core of the Earth is more dense than the Moon.
The giant impact hypothesis throughout recent months and years is coming under more serious investigation. The idea seems to falter in that--while the density of the lunar material is approximately the same as the Earth's outer material--the ratios of rare materials seem to be wrong (and there are other problems).
Essentially, the riddle of the Moon's origin continues to be debated. A plausible theory must somehow account for the Moon's close orbit, synchronized rotation, angular momentum, composition, and its large size relative to the Earth's.
While the giant impact hypothesis is perhaps the best of all, this theory of a raked-off Moon doesn't completely explain all of the mechanical and material makeup of Earth-Moon system.
The best theory to account for the origin of the Moon is probably the giant impact theory. However, in order to account for the Moon's origin, a sequence of the following processes is required:
Can it be possible that the processes required to create the Earth-Moon are reflective of the actions of a Creator rather than reflective of the results of a giant impact?
- A "raking-off" of Earth's outer material.
- The "formation" of a round Moon.
- A "fine-tuning" (to produce additional tilt of the Earth's axis).
- A "refinement" of the material makeup of the Moon (which is similar to the Earth's outer material--but not quite).
Review of functional composition
A primary example of functional composition concerns the angular sizes of the Sun and the Moon in that the Moon's apparent diameter--as viewed from the Earth--appears to have the same angular size as the Sun's diameter. Essentially, to an observer stationed on the Earth, both orbs appear to have an equal angular size--of about 0.5 degree. Of significance here is that equal angular sizes (of both the Sun and the Moon) are absolutely necessary to achieve the formation of total solar eclipses (and the rare formation of ring eclipses).
Another good example of functional design can be recited from the orbital movement of the Moon (which perpetually remains synchronized with the rotational rate). As shown in a section from above, the sidereal orbital period of the Moon around the Earth completes in 27.321666 days. To be specific, the rotation of the Moon is exactly synchronized with the sidereal period. So as the Moon rotates one time every 27.321666 days the sidereal period around the Earth is completed . . .and because both rates--the sidereal period and the rotation--are exactly the same, the Moon can be predicted to progress throughout its orbit with the same face of the Moon showing toward the Earth at all times.
The size and density of the Earth-Moon system, the Moon's close orbit, and the nearly circular orbit of the Moon--though beneficial in stabilizing Earth's tilt and spin--are also reflective of functional composition.
How can it be that a true planet the size of the Earth holds a relatively large, almost perfectly round Moon in its orbit? This close proximity of the Moon's orbit to the Earth cannot be explained through a capture process... as if the Moon were somehow captured into Earth's orbit. In fact, computer simulations indicate that the Earth isn't large enough to capture and hold a large Moon. (Even if the Moon were somehow captured and held, it is unlikely that a close circular orbit of the current angular momentum would result).
It seems evident from the cited equal angular sizes, synchronized spin and orbital rates, and close proximities that the Earth-Moon system does mirror a degree of Divine strategy in its design. In addition to these compositional characteristics, a bigger clue to a planned origin of the Earth and Moon comes from the rate of their traverse around the Sun. Of significance here is that interrelated time structures can be recognized from out of interfacing rates of solar days, synodic months, seasons, and solar years. Again, for additional information about interrelatedness of Earth-Moon-Sun cycles, refer to the following online publications:
________________________
The Genesis Record
A reader who has progressed thus far will probably wonder why a focus is now formed upon the biblical book of Genesis. After all, doesn't this book relate to a creation story that is not very scientific. The answer has to be 'yes' in the regard that the Genesis account of Divine creation in only 6 days doesn't square at all with fields of modern study. However, there is a most interesting flipside to the Genesis story in regard that the account happens to contain some jaw-dropping detail of perhaps the most UNUSUAL Earth-Moon-Sun conjunction that has ever been recorded.
To be more specific about an Earth-Moon conjunction detailed in Genesis, three chapters of this book (Chapters 6-8) have detail of a worldwide flood. The flood event is noted to have occurred throughout only one year of early human history, and is quite unusual in comparison with other ancient narratives.
What makes the flood story of Genesis so very unique is that the detail of some specific calendar dates (and related day counts) is given. Ultimately, a rather detailed chronology for the year of the great flood is tabled by the author of Genesis.
In addition to the flood narrative, the initial chapters of Genesis contain a story about the creation of the 1st man (Adam). While it appears that the very beginning chapter of Genesis may represent a poem, a rather significant genealogy is ultimately given for those persons who lived in the era from Adam to Noah.
Throughout the somewhat elaborate and lengthy chronology set forth in the rare Genesis record appear some rather peculiar calendar terms. Among these are: "the evening was and the morning was"; "40 days and 40 nights"; the celestial signs [owth or oth]; and the fixed times [mow`ed]. Also unique or unusual about the calendar or astronomical system referenced by this author is that the 17th or 27th day appears to be integral for dating certain events.
Other literature produced in the era of the Second Temple has very similar information to that shown in Genesis. The most comprehensive description of the flood (external to Genesis) can be recited from 'The Book of Jubilees'. An account of Noah and the flood is also given in 'Antiquities of the Jews' (by Flavius Josephus). The same flood account also appears in certain scrolls of the Sea Scroll library.
The various calendar dates shown, the corresponding day counts given, and the calendar terms used in Genesis make it possible to not only interpret the day and date of the flood but also to recognize that a combinational lunar and solar time track was within the knowledge of the astronomer-priest who wrote the initial chapters. Essentially, it seems very probable that a system of tracking both lunar and solar cycles was used to set down the chronology of Genesis.
Beginning with "the day that God created man", the author of Genesis was careful to present a genealogy for those patriarchs who came after Adam. The genealogical record of Genesis is significant in showing a specific time span (1656 years) from an epoch or beginning (at creation) to the year of the Deluge.
Of additional significance about the genealogy given for the patriarchs is that certain Jewish writers who flourished in the era of the Second Temple appear to have understood that the creation of the 1st man Adam and also the 1st day of the flood were events that both occurred on the (same) day of the vernal equinox.
This early-held belief about the Deluge arriving in sync with the spring season can clearly be recognized from writings attributed to the Jewish philosopher: Philo Judaeus (c. 25 BC - 45 AD). The commencement of the great flood on the first day of spring is manifest from a portion of his second dissertation on the book of Genesis--as follows:
"... the deluge fell on the day of the vernal equinox... the first man who was produced out of the earth was also created at the same season of the year, he whom the divine writer calls Adam... Since, therefore, the first beginning of the generation of our race, after the destruction caused by the deluge, commenced with Noah, men being again sown and procreated, therefore he also is recognised as resembling the first man born of the earth... putting them to shame because he would, unquestionably, never, after he had created the universe... have destroyed all the men who lived on the earth... if it had not been for the preposterous excess of their iniquities." (Questions and Answers on Genesis, Part 2:17).
The composite historical record does thus rather clearly indicate that the ancients believed Adam was created right on the day of the vernal equinox. After a time span of 1656 tropical years, the great flood was believed to have begun on this SAME DAY of the year cycle (on the first day of the spring season).
____________________________________ CHRONOLOGY OF THE DELUGE Equinox Alignments * ____________________________________ 1st day of Adam = 1st day of spring 1st day of Flood = 1st day of spring ____________________________________ *-The Deluge began on the equinox (the same day that Adam was created on).
So, a specific day and year for the flood event can be recognized from portions of early-written text. An epoch for the great flood is indicated right on the day of the vernal equinox and 1656 tropical years from "the day" of the vernal equinox when Adam was created.
A specific chronology of days and years can be additionally recognized from those portions of the Genesis record that have detail of a calendar count that spanned 40 days. This count is significant in the regard that--beginning with the first FULL DAY after the creation of Adam--the number of days across 1656 tropical years to the commencement of the flood (604840 days) can EXACTLY be divided into segments of 40 days (15121 cycles).
Thus, the author (or authors) of the Genesis record may have understood/interpreted cycles of 40 days in a specific order, or in a certain chronological sequence.
In substantiation of a hypothesis that the ancients held knowledge of 40 days--as a cyclical count--it seems to be rather significant that the limits of each passing tropical year can closely be determined by simply time tracking 40 days (in an unbroken loop). In fact, a perfect annual scribe can be achieved by time tracking this cycle in association with a 9-year cycle.
The cited calendar count of 40 days--prevalently referred to by early Hebrew writers--was almost surely used by ancient astronomers to augment an effective meter of the tropical year. The revolution of each tropical year can effectively be measured and metered out (on average) by simply keeping track of the renewal of 40 days in a loop cycle. For pertinent information concerning ancient astronomers and their indicated time track of a 9-year cycle, refer to the following online publication:
The possibility that early astronomers did interpret '40 days' relative to a cyclical count can be recited from various portions of Second-Temple literature. For example, the count of 40 days in a cycle can be recited from 'The Book of Jubilees'--as follows:
"... In the 1st week was Adam created... [His wife] in the 2nd week... And after Adam had completed 40 days... we brought him into the garden of Eden to till and keep it, but his wife they brought in on the 80th day... " (Jubilees 3: 8-10).
The cited quote is significant in the regard that primal priest-astronomers appear to have interpreted a calendar count of 40 days as commencing right on the 1st day of human history. Essentially, the 1st day of a running cycle (40 days) was interpreted in association with the day when the 1st man (Adam) was created.
The Genesis record likewise shows the commencement day of the great flood in association with a cyclical count of 40 days--as follows:
... [In the] 2nd renewal, the 17th day of the renewal, the SAME DAY were all the fountains of the great deep broken up, and the windows of heaven were opened. And the rain was upon the earth 40 days and 40 nights... " [Genesis 7:11].
On the basis of the day counts given, it can be deduced that the Genesis narrative agrees with other portions of period literature. For example, the first man (Adam) can be recited to have been created on the day of the vernal equinox (1 day prior to a 40 day count). In addition, the Deluge is shown to have commenced on the (same) day of the vernal equinox (on day 1 of a 40 day count). The Genesis record thus is recognizably the same, or similar, to other ancient accounts. [Note that the number of days across 1656 tropical years (from equinox-to-equinox) is exactly divisible into segments of 40 days.]
The indicated span of days from first full day in the life Adam to the day of the vernal equinox (after 1656 tropical years) is inherently equal to 604840 days. Because 604840 days can exactly be divided into cycles of 40 days then the cited day counts and calendar terms given for the creation of Adam, and for the day of the flood, do possibly stem from some common early-written source.
The references made by the author (or authors) of Genesis to a 40-day cycle are additionally significant in the regard that this respective time track exactly dovetails with a calendar count of 9 tropical years. A calendar interface can ultimately be recognized from the Genesis account of the flood because the given span of 1656 years can exactly be divided into calendar segments of 9 years each (184 complete cycles).
The indicated knowledge and use of an accurate calendar among the ancients is remarkable in the regard that a cycle of 9 years inherently times out together with a cycle of 40 days in a time span equal to 1656 tropical years. Again, more information about a calendar count of 9 years is contained in the following online publication:
Knowledge of a calendar count of 40 days in a loop cycle can additionally be detected amid the Genesis record of the Deluge. This respective count is shown relative to the commencement day of the flood--as cited. A count of 40 days is again shown after several more months in that same year cycle--as follows:
... [On the beginning day]... the rain was upon the earth 40 days and 40 nights... [The waters increased and then began to decrease until the 10th renewal]... and in the TEN on the ONE [= echad] to the renewal, were the tops of the mountains seen. And it came to pass at the end of 40 days... a raven... [and] a dove [were sent forth] to see if the waters were abated from off the face of the ground; But the dove... returned... for the waters were on the face of the whole earth... " [Genesis 7:11, 8: 5-9].
Because a count of 40 days is referenced at the beginning of the flood and again in the 10th renewal of the same year then it seems clear that the author (or authors) of Genesis might have held knowledge of 40 days as a calendar count.
An ancient Jewish writer (Philo Judaeus) made reference to the cited (Genesis) description of a 40-day cycle--as follows:
"... the overflow of the deluge took place for forty days... [thereafter] a hope of RENEWAL took place at intervals of forty days... " (Questions and Answers on Genesis, Part 2:33).
The count of 40 days as perhaps a cyclical count can also be recited from the book of Exodus--where it is shown that Moses was in the mount for 40 days and 40 nights (refer to Chapter 24: 10-18). The calendar term "40 days and 40 nights" is again recorded in the book of Deuteronomy, where Moses wrote:
"And I stayed in the mount ... to the 1st day, 40 days and 40 nights... " (refer to Chapter 10:10).
This passage shows "the 1st day" as immediately following 40 days and 40 nights. (Note that the Hebrew language usage of "rishown yowm"--or the beginning day--tends to indicate the track of a cyclical or a chronological count of 40 days).
Primal astronomer-priests thus appear to have understood 40 days as a cyclical count. It is here significant that Adam was believed to have been created right on the day of the vernal equinox with the 1st day after his creation in association with day 1 and year 1 of a calendar count of 40 days. The day of the flood appears to have likewise been understood to have commenced right on the day of the vernal equinox and in correspondence with day 1 and year 1 of the respective calendar count. Of additional significance is that a specific interval of days (604840 days) can be recognized from the content of those priestly records that pertain to the creation of Adam and to the epoch of the flood.
A given conclusion from the duplicate Hebrew record then is that the Earth was in the same orbital position around the annual transit of the Sun at both the beginning and also at the end of the cited span of time (at the turn of the vernal equinox).
___________________________________ CHRONOLOGY OF THE DELUGE Equinox Alignments * ___________________________________ Day 1 of Adam = 1st of spring 604840 days after = 1st of spring ___________________________________ *-The Deluge began on the equinox, 604840 days after Adam's first day
The day of the flood can also be related to a chronology of lunar months... and to a specific phase of the Moon's synodic return.
On the basis of the Genesis record, a Moon chronology appears to have began some 6 days prior to "the day that God created man". (Note that Adam wasn't believed to have been created until day 6 of creation week . . . with the 1st full day of his life on day 7 of the week).
Assuming then that early astronomer-priests did interpret the Moon; in symbol; to have stood with say its 1st day on the 1st day of the 1st week then the flood event would have likewise been interpreted to have occurred in coincidence with a SAME (or an identical) phase of the Moon.
____________________________________ MOON CHRONOLOGY (at Creation and at the Deluge) * ____________________________________ Day 1 of Creation = 1st of Month Day 1 of Deluge = 1st of Month ____________________________________ *-The Deluge came at the beginning of the lunar month (20482 periods as counted from creation day).
Note that 604840 days plus 6 more days totals 604846 days. In terms of cycles of the lunar month, a span of 604846 days inherently encompasses 20482 lunar molads. Essentially, if counted in association with the day of an initial lunar month, a count of 604846 days inherently ends in correspondence with a same day of a lunar month.
It here seems pertinent to note that the initial chapters of Genesis--written early in Temple Era--reflect a regard among members of the priesthood for the turn of the lunar quarter or the lunar week.
What is peculiar about accounting for the lunar week is that the priests appear to have tracked the unit of the lunar week in multiples of seven (or by sevens). Essentially, a tally or count of 7 lunar weeks (a pentecontad cycle) was succeeded by a subsequent count of 7 lunar weeks (the next pentecontad cycle). This unique count (7 lunar weeks) appears to have been endlessly performed. The cited cycle of 7 lunar weeks was probably tracked by primal astronomers for religious purposes and also to properly regulate the annual harvest. For pertinent information about the ancient track of 7 lunar weeks, refer to the following online publications:
A regard for tracking pentecontads among the Temple priests can especially be detected from Genesis chronology leading up to the day of the flood -- as follows:
Because the 1st day of creation week appears to have been interpreted in correspondence with the symbolic 1st day of the lunar month then the 1st day of the flood; by default; would likewise have been interpreted in coincidence with the 1st day of the lunar month.
Then, because the time span prior to the day of the flood can exactly be represented in units of the lunar month, this respective time span can also be represented in units of the lunar week (81928 lunar weeks).
It is here significant that the number of lunar weeks between the 1st day of creation week and the 1st day of the flood is inherently divisible by seven.
The indicated division of this time span in segments of 7 lunar weeks then points to the possibility that the priests also interpreted flood chronology in terms of the pentecontad cycle.
Note that the time span between the 1st day of creation week and the 1st day of the flood event can inherently be represented in units of 7 lunar weeks (11704 pentecontads). What is most remarkable is that this respective number of pentecontad cycles (11704 cycles) is yet again divisible by seven.
The Genesis record then points to the remarkable possibility that astronomer-priests in the era of the First Temple were keen to interpret the 1st day of creation week as the 1st day of a lunar-week unit. From this initial lunar week, a time sequence consisting of pentecontad cycles appears to have been interpreted prior to the 1st day of the flood.
The day of the great flood appears to have been understood by the priesthood as a day that did correspond with the renewal of a time cycle of 7 lunar weeks. Likewise, the duration of the flood appears to have been understood in terms of the pentecontad cycle. An analysis of Genesis chronology indicates that the flood lasted for an incredible duration of 7 pentecontads. Land travel did not commence again until the 27th day of the 2nd month--when the 50th lunar week was over.
Of further significance about the Moon's position at the Deluge is that Earth's rotational rate inherently conjoins with the same orbital phase of the Moon--every 49 synodic periods. Essentially, the length of 49 lunar months is also equal to the length of 1447 day units. (Note that the length of 7 sets of 7 lunar months, or 49 months, is exactly divisible by the length of the 24-hour day).
It is then most remarkable that the cited priest's record of a 1st day at the week of creation and a 1st day at the flood event can be recognized in terms of an identical Earth-Moon conjunction. In essence, on the basis of the day counts that are given in Genesis, the rotation of the Earth would have been identically conjoined (or aligned) with a same phase of the Moon on both of these occasions.
Note that the 1st man (Adam) is shown to have been created on the 6th day of creation week. Commencing with the 1st FULL DAY after the creation of Adam, the priest's record indicates a calendar count of 604840 days to the 1st day of the flood (as previously has been shown). Remarkable about the priestly record is that from the 1st day of creation week to the 1st day of the flood event is a time span that inherently is divisible by the length of 49 synodic periods (418 cycles). This respective span of time is also inherently divisible by a cycle of 1447 days (418 cycles) For pertinent information about a time cycle of 49 months, refer to the following online publication:
Thus, it seems to be significant that the priests would surely have held knowledge of a cycle of 49 Moons (or also 1447 rotations of the Earth). Knowledge of this conjunction cycle appears to clearly be mirrored in the Genesis record of a 1st day of creation week and a subsequent 1st day of a flood event.
_____________________________________ CHRONOLOGY OF THE DELUGE (Earth and Moon Alignments) * _____________________________________ 1st of 49 months = 1st of Creation 1st of 49-months = 1st of Deluge _____________________________________ * - Earth's spin and the period of the Moon do both align together every 49 months.
The fact that the Genesis record spans a lengthy time span (604846 days) and yet appears to be correct in its description of the alignment of the day of the flood with the synodic month and the tropical year is quite remarkable. The additional description of the day of the flood aligning with yet another day cycle (that of 40 days) can be interpreted as pretty amazing.
The Genesis record thus reflects that the priests might have held unusual--even advanced--knowledge of the spin and orbital rates. Essentially, an accurate method of tracking the spin and orbital movements would have been required to correctly predict that--after a number of days across a time span of over 1.5 millennia--the travel of the Moon would come again into alignment with the epoch of the vernal equinox!
The accurate Genesis record of lunisolar cycles timing out together on one specific day (the day of the flood) points to the clear possibility that the Genesis account could be authentic. (The record is at least authentic in terms of time cycles generated by the Earth and Moon). The authenticity of the Genesis story in this regard tends to at least indicate that advanced knowledge of astronomy must have been held by the primal priesthood.
The Temple priests appear to have been in possession of a literal set of records pertaining to a week of creation and also to a flood event--as cited. Unfortunately, the Genesis source doesn't fully explain which season of the year the great flood occurred in. However, certain writings attributed to the patriarch Enoch are explicit in showing that the vernal equinox was understood as the beginning of the year cycle, and writings attributed to Philo Judaeus are also explicit in stating that "the deluge fell on the day of the vernal equinox... ".
For additional information about time cycles recorded in passages of Temple-Era literature, refer to the following online publications:
________________________
APPENDIX A
EXCESS OF THE DURATION OF THE DAY TO 86400S AND
ANGULAR VELOCITY OF THE EARTH'S ROTATION
(SINCE 1623)
Data from IERS
Mean annual values of the duration of the day D, are available for the last four centuries. For the interval 1623-1955, the data are those provided by L.V. Morrison, Royal Greenwich Observatory, interpolated for the middle of the year. The mean solar time has been referred to the dynamical time scale derived from the time argument of the lunar ephemeris.
The duration of the day has been obtained:
- from 1623 to 1860, by derivative of cubic splines fitted on individual values of the difference between mean solar time and dynamical time (13 knots),
- from 1861 to 1955, by a 5-point quadratic convolute.
More information on the computation of the duration of the day is available in Stephenson and Morrison (1984), with an estimation of the accuracy of these evaluations.
From 1956 up to present, the duration of the day has been obtained from the BIH/IERS values of UT1-TAI ; the table gives annual averages. At the level of precision of these values of the duration of the day, the unit of the dynamical time and the unit of TAI can be considered as having the same duration. Thus D is expressed in present SI units. The table gives also the values of the angular velocity of the Earth's rotation w derived from the listed values of D.
A table showing mean annual values for the duration of the day (1623 to 1955) is shown in the following online publication:
________________________
APPENDIX B
Universal Time and Delta-T
Atomic Time, with the unit of duration the Systeme International (SI) second defined as the duration of 9,192,631,770 cycles of radiation corresponding to the transition between two hyperfine levels of the ground state of cesium 133.
TAI is the International Atomic Time scale, a statistical timescale based on a large number of atomic clocks.
Universal Time (UT) is counted from 0 hours at midnight, with unit of duration the mean solar day, defined to be as uniform as possible despite variations in the rotation of the Earth.
UT0 is the rotational time of a particular place of observation. It is observed as the diurnal motion of stars or extraterrestrial radio sources.
UT1 is computed by correcting UT0 for the effect of polar motion on the longitude of the observing site. It varies from uniformity because of the irregularities in the Earth's rotation.
Coordinated Universal Time (UTC) differs from TAI by an integral number of seconds. UTC is kept within 0.9 seconds of UT1 by the introduction of one-second steps to UTC, the "leap second." To date these steps have always been positive.
Dynamical Time replaced ephemeris time as the independent argument in dynamical theories and ephemerides. Its unit of duration is based on the orbital motions of the Earth, Moon, and planets.
Terrestrial Time (TT), (or Terrestrial Dynamical Time, TDT), with unit of duration 86400 SI seconds on the geoid, is the independent argument of apparent geocentric ephemerides. TDT = TAI + 32.184 seconds.
Barycentric Dynamical Time (TDB), is the independent argument of ephemerides and dynamical theories that are referred to the solar system barycenter. TDB varies from TT only by periodic variations.
Geocentric Coordinate Time (TCG) is a coordinate time having its spatial origin at the center of mass of the Earth. TCG differs from TT as: TCG - TT = Lg x (JD -2443144.5) x 86400 seconds, with Lg = 6.969291e-10.
Barycentric Coordinate Time (TCB)is a coordinate time having its spatial origin at the solar system barycenter. TCB differs from TDB in rate. The two are related by: TCB - TDB = iLb x (JD -2443144.5) x 86400 seconds, with Lb = 1.550505e-08.
Delta-T = (TDT-UT).
________________________
APPENDIX C
Value of Delta T
Table and Text--as shown below--borrowed from
NASA Eclipse Web Site
As Earth rotates on its axis, tidal friction is imposed on it through the gravitational attraction with the Moon and, to a lesser extent, the Sun. This secular acceleration gradually transfers angular momentum from Earth to the Moon. As Earth loses energy and slows down, the Moon gains this energy and its orbital period and distance from Earth increase.
R. F. Stephenson and collaborators have produced a number of seminal works in the field of Earth's rotation over the past several millennia. In particular, they have identified hundreds of eclipse and occultation observations in early European, Middle Eastern and Chinese annals, manuscripts, canons and records. In spite of their relatively low precision, these data represent our only record to the value of delta-T during the past several millennia.
In Atlas of Historical Eclipse Maps East Asia 1500 BC - AD 1900, Stephenson and Houlden (1986) present two empirically derived expressions to describe the behavior of delta-T prior to telescopic records (pre-1600):
(1) prior to 948 AD
delta-T (seconds) = 1830 - 405*t + 46.5*t^2
(t = centuries since 948 AD)
(2) 948 AD to 1600 AD
delta-T (seconds) = 22.5*t^2
(t = centuries since 1850 AD)
More recently, Stephenson presents a new analysis of most if not all known solar and lunar eclipses that occurred during the period -700 to +1600 (Historical Eclipses and Earth's Rotation, 1997). The new analysis uses a spline to fit the observations.
The following table lists values of delta-T (seconds) derived from Stephenson and Houlden (1986), along with Stephenson (1997) for comparison.
Year delta-T delta-T
(1986) (1997)
-2000 54181 -
-1900 51081 -
-1800 48073 -
-1700 45159 -
-1600 42338 -
-1500 39610 -
-1400 36975 -
-1300 34433 -
-1200 31984 -
-1100 29627 -
-1000 27364 -
-900 25194 -
-800 23117 -
-700 21133 -
-600 19242 -
-500 17444 16800
-400 15738 15300
-300 14126 14000
-200 12607 12800
-100 11181 11600
0 9848 10600
100 8608 9600
200 7461 8600
300 6406 7700
400 5445 6700
500 4577 5700
600 3802 4700
700 3120 3800
800 2531 3000
900 2035 2200
1000 1625 1600
1100 1265 1100
1200 950 750
1300 680 470
1400 455 300
1500 275 180
1600 140 110
(all values in seconds)
References for Delta-T
- Morrison, L.V. and Ward, C. G., "An analysis of the transits of Mercury: 1677-1973", Mon. Not. Roy. Astron. Soc., 173, 183-206, 1975.
- Stephenson F.R and Houlden M.A., Atlas of Historical Eclipse Maps: East Asia 1500 BC - AD 1900, Cambridge Univ.Press., 1986.
- Stephenson F.R., Historical Eclipses and Earth's Rotation , Cambridge Univ.Press, 1997.
________________________
APPENDIX D
The Coral Record
Fossilized corals indicate that Earth's spin was faster in the distant past. For more information, please refer to the following online publication:
________________________
APPENDIX E
Exploring the Slowing Rate of the
Rotation of the Earth
By: James D. Dwyer
The following guide will illustrate the significance of the slowing spin of the Earth. This slowing spin rate directly relates to the definition of the synodic month and the solar year of the past--where the number of days in previous month and year cycles has become reduced throughout time.
This does not suggest that the movement of the Earth in its orbit completes at a significantly slower rate, but rather that the spin-rate relative to the orbital rate could have changed. Essentially, the solar year of the past may have contained a higher rate of day counts than the current solar year. (Note that the Earth was determined to spin about 0.0017 seconds slower across the twentieth century).
It is convenient to explore previous relationships through the use of a simple constant rate. (This small constant rate represents the average number of seconds per century by which the length of the day is gradually increasing).
The use of an average constant rate for the increasing length of the day makes a ballpark determination of time changes easy to perform (and this rather low level of accuracy may be completely good enough for testing ideas, and for exploring ranges and limits).
Also, the use of a simple constant rate for an increasing length of the day makes the effect of plausible time changes clear and easy to understand.
Example 1 -- Assume that the length of the day is increasing at the ballpark estimate of 0.001 seconds per century. Based upon this rough conservative estimate then how many days were in the annual cycle at six-thousand in the past?
A solution for this type of problem might be thought through as follows:
(1) Because Earth's spin loses 0.001 seconds each century, and because the rate of the solar year is 365.24 days then the definition of the solar year is changing at the rate of 0.3652 spin-seconds for each century.
(2) The number of centuries elapsed throughout the previous 6000 years are equal to 60 centuries.
(3) This set of assumptions and parameters then indicates that the definition of the solar year was 21.9 spin-seconds faster at 60 centuries. (Note that 60 times 0. 3652 spin-seconds equals a change of 21.91 spin-seconds).
Example 2 -- Assume that the length of the day is increasing at an estimated 0.001 seconds per century. Based upon this conservative estimate then when in the past did the annual cycle complete in 365.2442 days?
A solution for this type of problem might be thought through as follows:
(1) Because Earth's spin loses 0.001 seconds each century, and because the rate of the solar year is 365.24 days then the definition of the solar year is changing at the rate of 0.3652 spin-seconds for each century.
(2) The target rate of the solar year (365.2442 days) minus the current rate (365.2422 days) is equal to a net change of 181.44 spin-seconds.
(3) This set of assumptions and parameters then indicates that at 500.5 centuries ago the solar year was equal to 365.2442 solar days. (Note 181.44 spin-seconds divided by 0.3625 seconds per century is equal to 500.5 centuries).
________________________
Copyright © 2007-2021 A-Quest-For-Creation-Answers
________________________
RELATED READING
An Interrelated System A Time Count of 360 Days The Moon as a Time Meter Functional Time Design The Jubilee Time Cycle Significance of 40 Days Ancient Astronomy Portals or Annual Gates Circle-of-Seven Significant Lunar Week Slowing Spin of the Earth Access other articlesPlease feel free to download and distribute--but not sell--the articles and booklets listed above. (Note that the published material is subject to constant revision. Be advised that corrections, amendments, and new interpretations are frequently made.)
________________________
<Return to Home Page>